Advertisements
Advertisements
Question
In figure, if ∠1 = ∠2 and ΔNSQ ≅ ΔMTR, then prove that ΔPTS ~ ΔPRQ.
Solution
According to the question,
ΔNSQ ≅ ΔMTR
∠1 = ∠2
Since,
∆NSQ = ∆MTR
So,
SQ = TR ...(i)
Also,
∠1 = ∠2 ⇒ PT = PS ...(ii) [Since, sides opposite to equal angles are also equal]
From equations (i) and (ii),
`("PS")/("SQ") = ("PT")/("TR")`
⇒ ST || QR
By converse of basic proportionality theorem, If a line is drawn parallel to one side of a triangle to intersect the other sides in distinct points, the other two sides are divided in the same ratio.
∴ ∠1 = PQR
And
∠2 = ∠PRQ
In ∆PTS and ∆PRQ,
∠P = ∠P ...[Common angles]
∠1 = ∠PQR ...(Proved)
∠2 = ∠PRQ ...(Proved)
∴ ∆PTS – ∆PRQ ...[By AAA similarity criteria]
Hence proved.
APPEARS IN
RELATED QUESTIONS
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
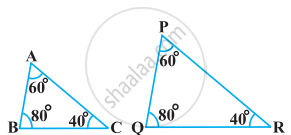
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
In the following figure, altitudes AD and CE of ΔABC intersect each other at the point P. Show that:
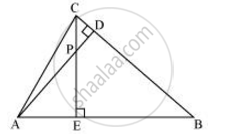
ΔAEP ∼ ΔCDP
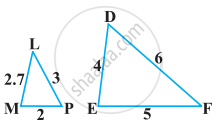
The sides of certain triangles are given below. Determine which of them right triangles are.
1.6cm, 3.8cm, 4cm
If ΔABC ~ ΔEDF and ΔABC is not similar to ΔDEF, then which of the following is not true?
In figure, BD and CE intersect each other at the point P. Is ΔPBC ~ ΔPDE? Why?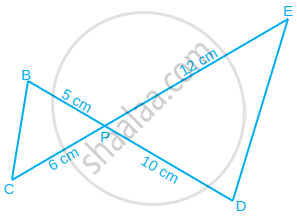
D is a point on side QR of ΔPQR such that PD ⊥ QR. Will it be correct to say that ΔPQD ~ ΔRPD? Why?
If in two triangles ABC and PQR, `(AB)/(QR) = (BC)/(PR) = (CA)/(PQ)`, then ______.
In figure, two line segments AC and BD intersect each other at the point P such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30°. Then, ∠PBA is equal to ______.
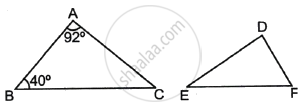
If ΔABC ∼ ΔDEF such that ∠A = 92° and ∠B = 40°, then ∠F = ?