Advertisements
Advertisements
Question
In Δ ABC, the perpendicular bisector of AB and AC meet at 0. Prove that O is equidistant from the three vertices. Also, prove that if M is the mid-point of BC then OM meets BC at right angles.
Solution
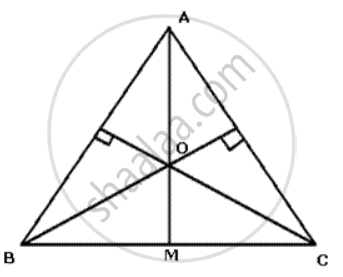
Since O lies on the perpendirular bisector of AB, O is equidistant from A and B.
OA = OB ........ (i)
Again, O lies on the perpendirular bisector of AC, O is equidistant from A and C.
OA = OC ......... (ii)
From (i) and (ii)
OB= OC
Now in Δ OBM and Δ OCM,
OB = OC (proved)
OM=OM
BM =CM ( M is mid-point of BC)
Therefore, Δ OBM and Δ OCM are congruent.
∠ OMB= ∠ OMC
But BMC is a straight line, so
∠ OMB =∠ OMC = 90°
Thus, OM meets BC at right angles.
APPEARS IN
RELATED QUESTIONS
Construct a right angled triangle PQR, in which ∠Q = 90°, hypotenuse PR = 8 cm and QR = 4.5 cm. Draw bisector of angle PQR and let it meets PR at point T. Prove that T is equidistant from PQ and QR.
In triangle LMN, bisectors of interior angles at L and N intersect each other at point A. Prove that:
- Point A is equidistant from all the three sides of the triangle.
- AM bisects angle LMN.
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
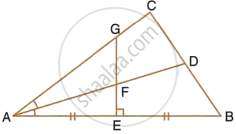
F is equidistant from A and B.
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
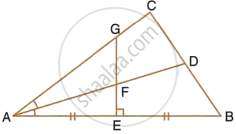
F is equidistant from AB and AC.
In the given triangle ABC, find a point P equidistant from AB and AC; and also equidistant from B and C.
Describe the locus of the door handle, as the door opens.
Describe the locus of points at distances less than 3 cm from a given point.
By actual drawing obtain the points equidistant from lines m and n; and 6 cm from a point P, where P is 2 cm above m, m is parallel to n and m is 6 cm above n.
ΔPBC, ΔQBC and ΔRBC are three isosceles triangles on the same base BC. Show that P, Q and R are collinear.
Given: ∠BAC, a line intersects the arms of ∠BAC in P and Q. How will you locate a point on line segment PQ, which is equidistant from AB and AC? Does such a point always exist?
