Advertisements
Advertisements
Question
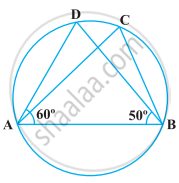
In the following figure, if ∠DAB = 60º, ∠ABD = 50º, then ∠ACB is equal to ______.
Options
60º
50º
70º
80º
Solution
In the following figure, if ∠DAB = 60º, ∠ABD = 50º, then ∠ACB is equal to 70º.
Explanation:

Given: ∠DAB = 60°, ∠ABD = 50°
Using the theorem “angles in the same segment of the circle are equal”:
∴ ∠ADB = ∠ACB ...(i)
In triangle ABD, using the angle sum property of triangle, we have:
∠ABD + ∠ADB + ∠DAB = 180°
⇒ 50° + ∠ADB + 60° = 180°
⇒ ∠ADB = 180° – 50° – 60°
⇒ ∠ADB = 70°
From equation (i), we have:
∴ ∠ACB = 70°
APPEARS IN
RELATED QUESTIONS
If ΔABC is isosceles with AB = AC and C (0, 2) is the in circle of the ΔABC touching BC at L, prove that L, bisects BC.
In the following figure, AB is the diameter of a circle with centre O and CD is the chord with length equal to radius OA.
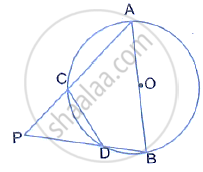
Is AC produced and BD produced meet at point P; show that ∠APB = 60°
In the given figure, a cradle inscribed in a triangle ABC touches the sides AB, BC and CA at points D, E and F respectively. If AB = 14cm, BC = 8cm and CA=12 cm. Find the length AD, BE and CF.

In the given figure, ΔABC is an equilateral triangle. Find m∠BEC.

In the given figure, a ∆ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC are of lengths 8 cm and 6 cm respectively. Find the lengths of sides AB and AC, when area of ∆ABC is 84 cm2.

In the given figure, chord EF || chord GH. Prove that, chord EG ≅ chord FH. Fill in the blanks and write the proof.

ABC is a right triangle in which ∠B = 90°. If AB = 8 cm and BC = 6 cm, find the diameter of the circle inscribed in the triangle.
Find the missing values in the following table for the circles with radius (r), diameter (d) and Circumference (C).
| radius (r) | diameter (d) | Circumference (C) |
| 1760 cm |
All the radii of a circle are _______________
A line segment with its end points on the circle is called a ______________
