Advertisements
Advertisements
Question
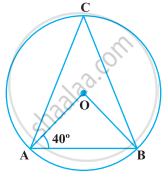
In the following figure, if ∠OAB = 40º, then ∠ACB is equal to ______.
Options
50º
40º
60º
70°
Solution
In the following figure, if ∠OAB = 40º, then ∠ACB is equal to 50º.
Explanation:

Given: ∠OAB = 40º
Now, in triangle OAB,
OA = OB ...[Radii of circle]
So, ∠OAB = ∠OBA = 40º ...[Angle opposite to equal sides are equal]
So, ∠AOB = 180º – (40º + 40º) = 100º
As we know that angle subtended by an arc of a circle at the center is double the angle subtended by it at any point on the remaining part of the circle.
So, ∠ACB = `1/2` ∠AOB = `1/2 xx 100^circ` = 50º
APPEARS IN
RELATED QUESTIONS
In Figure 1, common tangents AB and CD to the two circles with centres 01and 02 intersect at E. Prove that AB = CD.

In the given figure, PQ is a chord of length 8cm of a circle of radius 5cm. The tangents at P and Q intersect at a point T. Find the length TP

PA and PB are tangents from P to the circle with centre O. At point M, a tangent is drawn cutting PA at K and PB at N. Prove that KN = AK + BN.
In the below fig. O is the centre of the circle. If ∠APB = 50°, find ∠AOB and ∠OAB.

In the given figure, chords AD and BC intersect each other at right angles at a point P. If ∠DAB = 35°, then

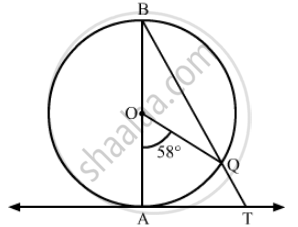
In the given figure, AB is a diameter of a circle with centre O and AT is a tangent. If \[\angle\] AOQ = 58º, find \[\angle\] ATQ.
Choose correct alternative answer and fill in the blank.
Radius of a circle is 10 cm and distance of a chord from the centre is 6 cm. Hence the length of the chord is .........
ABC is a triangle with AB = 10 cm, BC = 8 cm and AC = 6 cm (not drawn to scale). Three circles are drawn touching each other with the vertices as their centres. Find the radii of the three circles.

The ______________ is the longest chord of a circle
A quadrilateral ABCD is inscribed in a circle such that AB is a diameter and ∠ADC = 130º. Find ∠BAC.
