Advertisements
Advertisements
Question
In the given figure, ΔABC is an equilateral triangle. Find m∠BEC.

Solution
It is given that, Δ ABC is an equilateral triangle
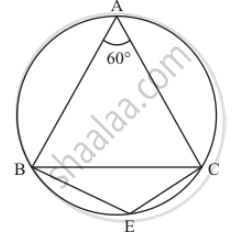
We have to find `m angle BEC`
Since Δ ABC is an equilateral triangle.
So `angle A + angle B + angle C = 180°`
And
`angle A = angleB = angleC` = 60° …… (1)
Since, quadrilateral BACE is a cyclic qualdrilateral
So , `angle A + angleE = 180°` (Sum of opposite angles of cyclic quadrilateral is 180° .)
`60° + angle E = 180°`
`angle E = 120°`
Hence
`m angle BEC = 120°`
APPEARS IN
RELATED QUESTIONS
In the given figure, PQ is chord of a circle with centre O an PT is a tangent. If
∠QPT = 60°, find the ∠PRQ.

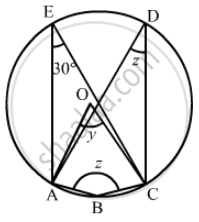
In the given figure, O is the centre of the circle. If ∠CEA = 30°, Find the values of x, y and z.
If the length of a chord of a circle is 16 cm and is at a distance of 15 cm from the centre of the circle, then the radius of the circle is
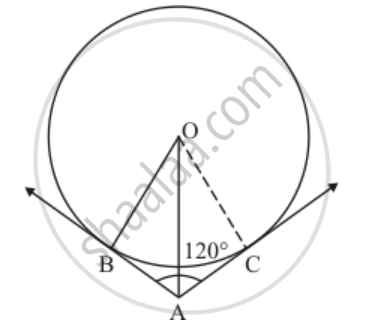
In the given figure, two tangents AB and AC are drawn to a circle with centre O such that ∠BAC = 120°. Prove that OA = 2AB.
In following fig. ABC is an equilateral triangle . A circle is drawn with centre A so that ot cuts AB and AC at M and N respectively. Prove that BN = CM.

Use the figure given below to fill in the blank:
EF is a ______ of the circle.

Construct a triangle XYZ in which XY = YZ= 4.5 cm and ZX = 5.4 cm. Draw the circumcircle of the triangle and measure its circumradius.
Can the length of a chord of a circle be greater than its diameter ? Explain.
In the following figure, O is the centre of the circle, ∠BCO = 30°. Find x and y.

A circle of radius 3 cm with centre O and a point L outside the circle is drawn, such that OL = 7 cm. From the point L, construct a pair of tangents to the circle. Justify LM and LN are the two tangents.
