Advertisements
Advertisements
Question
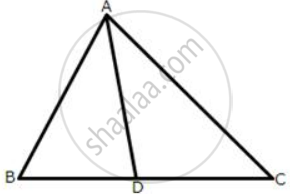
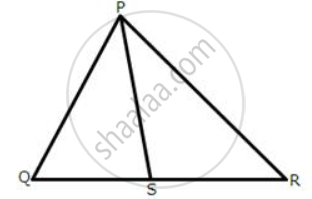
In the following figures, the sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and median PS of the triangle PQR.
Prove that ΔABC and ΔPQR are congruent.
 |
 |
Solution
Given: AB = PQ ; BC = QR ; AD = PS
To prove: ΔABC ≅ ΔPQR
Proof:
BC = QR
2 BD = 2 QS
BD = QS ...(i)
In Δ ABD and Δ PQS
AB = PQ ...[Given]
BD = QS ...[From equation (i)]
AD = PS ...[Given]
∴ ΔABD ≅ ΔPQS ...[by SSS rule]
Then, ∠B = ∠Q ...[by CPCTC] ...(ii)
In ΔABC and ΔPQR
AB = PQ ...[Given]
∠B = ∠Q ...[ii]
BC = QR ...[Given]
∴ ΔABC ≅ ΔPQR ...[by SAS rule]
Hence proved.
APPEARS IN
RELATED QUESTIONS
Which congruence criterion do you use in the following?
Given: AC = DF
AB = DE
BC = EF
So, ΔABC ≅ ΔDEF

Which congruence criterion do you use in the following?
Given: ZX = RP
RQ = ZY
∠PRQ = ∠XZY
So, ΔPQR ≅ ΔXYZ

In two triangles ABC and DEF, it is given that ∠A = ∠D, ∠B = ∠E and ∠C =∠F. Are the two triangles necessarily congruent?
In the given figure, ABC is an isosceles triangle whose side AC is produced to E. Through C, CD is drawn parallel to BA. The value of x is

D, E, F are the mid-point of the sides BC, CA and AB respectively of ΔABC. Then ΔDEF is congruent to triangle
If AP bisects angle BAC and M is any point on AP, prove that the perpendiculars drawn from M to AB and AC are equal.
From the given diagram, in which ABCD is a parallelogram, ABL is a line segment and E is mid-point of BC.
prove that : AL = 2DC
In the figure, given below, triangle ABC is right-angled at B. ABPQ and ACRS are squares. 
Prove that:
(i) ΔACQ and ΔASB are congruent.
(ii) CQ = BS.
In the following diagram, ABCD is a square and APB is an equilateral triangle.
(i) Prove that: ΔAPD ≅ ΔBPC
(ii) Find the angles of ΔDPC.
In quadrilateral ABCD, AD = BC and BD = CA.
Prove that:
(i) ∠ADB = ∠BCA
(ii) ∠DAB = ∠CBA
