Advertisements
Advertisements
Question
In the figure, given below, triangle ABC is right-angled at B. ABPQ and ACRS are squares. 
Prove that:
(i) ΔACQ and ΔASB are congruent.
(ii) CQ = BS.
Solution
Given: A(Δ ABC) is right-angled at B.
ABPQ and ACRS are squares
To Prove:
(i) ΔACQ ≅ ΔASB
(ii) CQ = BS
Proof:
(i)
∠ QAB = 90° ...[ ABPQ is a square ] ...(1)
∠ CAS = 90° ...[ ACRS is a square ] ...(2)
From (1) and (2) , We have
∠ QAB = ∠CAS ...(3)
Adding ∠BAC to both sides of (3), We have
∠ QAB + ∠BAC = ∠CAS+ ∠BAC
⇒ ∠QAC = ∠BAS ...(4)
In ΔACQ ≅ ΔASB, (by SAS)
QA = AB ...[ Sides of a square ABPQ ]
∠QAC = ∠SAB ...[ From(4) ]
AC = AS ...[ sides of a square ACRS ]
∴ By Side -Angle-Side criterion of congruence,
ΔACQ ≅ ΔASB
(ii)
The corresponding parts of the congruent triangles are congruent,
∴ CQ = SB ...[ c.p.c.t. ]
APPEARS IN
RELATED QUESTIONS
In quadrilateral ACBD, AC = AD and AB bisects ∠A (See the given figure). Show that ΔABC ≅ ΔABD. What can you say about BC and BD?

In Fig. 10.99, AD ⊥ CD and CB ⊥. CD. If AQ = BP and DP = CQ, prove that ∠DAQ = ∠CBP.

Prove that the perimeter of a triangle is greater than the sum of its altitudes.
In two congruent triangles ABC and DEF, if AB = DE and BC = EF. Name the pairs of equal angles.
In two triangles ABC and ADC, if AB = AD and BC = CD. Are they congruent?
D, E, F are the mid-point of the sides BC, CA and AB respectively of ΔABC. Then ΔDEF is congruent to triangle
If the following pair of the triangle is congruent? state the condition of congruency:
In ΔABC and ΔPQR, AB = PQ, AC = PR, and BC = QR.
The perpendicular bisectors of the sides of a triangle ABC meet at I.
Prove that: IA = IB = IC.
In the given figure: AB//FD, AC//GE and BD = CE;
prove that:
- BG = DF
- CF = EG
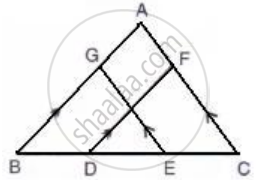
In the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that : ED = EF

