Advertisements
Advertisements
Question
ABCD is a parallelogram. The sides AB and AD are produced to E and F respectively, such produced to E and F respectively, such that AB = BE and AD = DF.
Prove that: ΔBEC ≅ ΔDCF.
Solution
ABCD is a parallelogram, The sides AB and AD are produced to E and F respectively,
such that AB = BE and AD = DF
We need to prove that ΔBEC ≅ ΔDCF.

Proof:
AB = DC ...[ Opposite sides of a parallelogram ] ...(1)
AB = BE ...[ Given ] ...(2)
From (1) and (2), We have
BE = DC ...(3)
AD = BC ...[ Opposite sides of a parallelogram ] ...(4)
AD = DF ....[Given] ...(5)
From (4) and (5), we have
BC = DF ...(6)
Since AD II BC, the corresponding angles are equal.
∴ ∠DAB = ∠CBE ...(7)
Since AB II DC, the corresponding angles are equal.
∴ ∠DAB = ∠FDC ...(8)
From (7) and (8), we have
∠CBE = ∠FDC
ln ΔBEC and ΔDCF
BF = DC ....[ from (3) ]
∠CBE = ∠FDC ...[ from (9) ]
BC = DF ....[ from (6) ]
∴ By Side-Angle-Side criterion of congruence,
ΔBEC ≅ ΔDCF
Hence proved.
APPEARS IN
RELATED QUESTIONS
In quadrilateral ACBD, AC = AD and AB bisects ∠A (See the given figure). Show that ΔABC ≅ ΔABD. What can you say about BC and BD?

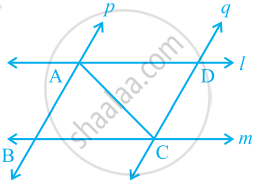
l and m are two parallel lines intersected by another pair of parallel lines p and q (see the given figure). Show that ΔABC ≅ ΔCDA.
In the given figure, AC = AE, AB = AD and ∠BAD = ∠EAC. Show that BC = DE.

Which of the following statements are true (T) and which are false (F):
Two right triangles are congruent if hypotenuse and a side of one triangle are respectively equal equal to the hypotenuse and a side of the other triangle.
If the following pair of the triangle is congruent? state the condition of congruency :
In Δ ABC and Δ DEF, AB = DE, BC = EF and ∠ B = ∠ E.
From the given diagram, in which ABCD is a parallelogram, ABL is a line segment and E is mid-point of BC.
Prove that:
(i) ΔDCE ≅ ΔLBE
(ii) AB = BL.
(iii) AL = 2DC
In the given figure, AB = DB and Ac = DC.

If ∠ ABD = 58o,
∠ DBC = (2x - 4)o,
∠ ACB = y + 15o and
∠ DCB = 63o ; find the values of x and y.
In the parallelogram ABCD, the angles A and C are obtuse. Points X and Y are taken on the diagonal BD such that the angles XAD and YCB are right angles.
Prove that: XA = YC.
In the figure, given below, triangle ABC is right-angled at B. ABPQ and ACRS are squares. 
Prove that:
(i) ΔACQ and ΔASB are congruent.
(ii) CQ = BS.
In a triangle, ABC, AB = BC, AD is perpendicular to side BC and CE is perpendicular to side AB.
Prove that: AD = CE.
