Advertisements
Advertisements
Question
In the parallelogram ABCD, the angles A and C are obtuse. Points X and Y are taken on the diagonal BD such that the angles XAD and YCB are right angles.
Prove that: XA = YC.
Solution
ABCD is a parallelogram in which ∠A and ∠C are obtuse.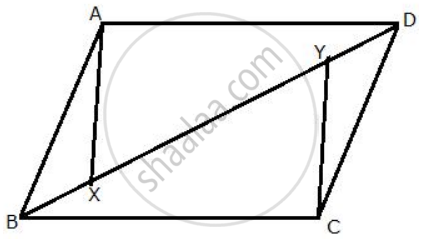
Points X and Y are taken on the diagonal BD.
Such that ∠XAD = ∠YCB = 90°.
We need to prove that XA = YC
Proof:
ln ΔXAD and ΔYCB
∠XAD = ∠YCB= 90° ...[ Given ]
AD = BC ...[ Opposite sides of a parallelogram ]
∠ADX = ∠CBY ...[ Alternate angles ]
∴ By Angle-Side-Angle criterion of congruence,
ΔXAD ≅ ΔYCB
The corresponding parts of the congruent triangles are congruent.
∴ XA = YC ...[ c.p.c.t. ]
Hence proved.
APPEARS IN
RELATED QUESTIONS
AD and BC are equal perpendiculars to a line segment AB (See the given figure). Show that CD bisects AB.

Which congruence criterion do you use in the following?
Given: ZX = RP
RQ = ZY
∠PRQ = ∠XZY
So, ΔPQR ≅ ΔXYZ

If the following pair of the triangle is congruent? state the condition of congruency :
In Δ ABC and Δ DEF, AB = DE, BC = EF and ∠ B = ∠ E.
Use the information in the given figure to prove:
- AB = FE
- BD = CF
In a triangle ABC, D is mid-point of BC; AD is produced up to E so that DE = AD.
Prove that :
(i) ΔABD and ΔECD are congruent.
(ii) AB = CE.
(iii) AB is parallel to EC
A line segment AB is bisected at point P and through point P another line segment PQ, which is perpendicular to AB, is drawn. Show that: QA = QB.
From the given diagram, in which ABCD is a parallelogram, ABL is a line segment and E is mid-point of BC.
Prove that:
(i) ΔDCE ≅ ΔLBE
(ii) AB = BL.
(iii) AL = 2DC
In quadrilateral ABCD, AD = BC and BD = CA.
Prove that:
(i) ∠ADB = ∠BCA
(ii) ∠DAB = ∠CBA
In the following figure, AB = EF, BC = DE and ∠B = ∠E = 90°.
Prove that AD = FC.
ABC is an isosceles triangle with AB = AC and BD and CE are its two medians. Show that BD = CE.
