Advertisements
Advertisements
Question
Nazima is fly fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the road. Assuming that her string (from the tip of her road to the fly) is taut, how much string does she have out (in the given figure)? If she pulls the string at the rate of 5 cm per second, what will the horizontal distance of the fly from her after 12 seconds.

Solution
Let us take
AB = Tip of fishing rod above the surface of the water
BC = The string rest from directly under the tip of the rod.
AC = The length of string

In ABC right triangle right angled at B
`AC^2=AB^2+BC^2`
`AC^2=1.8^2+2.4^2`
`AC^2=3.24+5.76`
`AC^2=9`
`AC^2=sqrt9`
`AC^2sqrt(3xx3)`
Hence 3 m string is out
The string pulled in 12 seconds is equal to `12xx5=60cm=0.6m` to point D
In this case the diagram will look like the following

Now The length of the new string AD = AC − CD = 3.00 − 0.6 = 2.4 m
Now in triangle ADB we have
`1.8^2+BD^2=2.4^2`
`3.24+BD^2=5.76`
`BD^2=5.76-3.24`
`BD^2=2.52`
`BD=1.587`
∴ Required distance = `1.587+1.2=2.79 m`
Hence, the horizontal distance is `2.97m.`
APPEARS IN
RELATED QUESTIONS
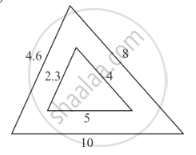
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
In ∆ABC, D and E are points on sides AB and AC respectively such that AD ✕ EC = AE ✕ DB. Prove that DE || BC.
Prove that in an equilateral triangle, three times the square of a side is equal to four times the square of its altitudes.
In ∆ABC, given that AB = AC and BD ⊥ AC. Prove that BC2 = 2 AC. CD
In a quadrilateral ABCD, ∠B = 90°. If AD2 = AB2 + BC2 + CD2 then prove that ∠ACD = 90°.
State AAA similarity criterion.
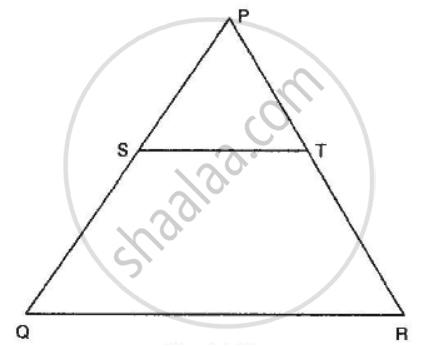
In the given figure, S and T are points on the sides PQ and PR respectively of ∆PQR such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ∆PST and ∆PQR.
∆ABC is such that AB = 3 cm, BC = 2 cm and CA = 2.5 cm. If ∆DEF ∼ ∆ABC and EF = 4 cm, then perimeter of ∆DEF is
In ∆ABC, a line XY parallel to BC cuts AB at X and AC at Y. If BY bisects ∠XYC, then
In a right triangle ABC right-angled at B, if P and Q are points on the sides AB and AC respectively, then
