Advertisements
Advertisements
Question
PQRS is a parallelogram. T is the mid-point of PQ and ST bisects ∠PSR.
Prove that: ∠RTS = 90°
Solution

∠PST = ∠TSR
∠QRT = ∠TRS
∠QRS + ∠PSR = 180° ...(adjacent angles of || gm are supplementary)
Multiplying by `(1)/(2)`
`(1)/(2)∠"QRS" + (1)/(2)∠"PSR" = (1)/(2) xx x180°`
∠TSR + ∠TRS = 90°
In ΔSTR,
∠TSR + ∠RTS + ∠TRS = 180°
90° + ∠RTS = 180°
∠RTS = 90°.
APPEARS IN
RELATED QUESTIONS
E is the mid-point of side AB and F is the mid-point of side DC of parallelogram ABCD. Prove that AEFD is a parallelogram.
The diagonal BD of a parallelogram ABCD bisects angles B and D. Prove that ABCD is a rhombus.
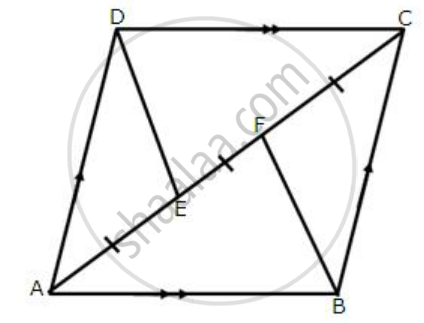
The alongside figure shows a parallelogram ABCD in which AE = EF = FC.
Prove that:
- DE is parallel to FB
- DE = FB
- DEBF is a parallelogram.
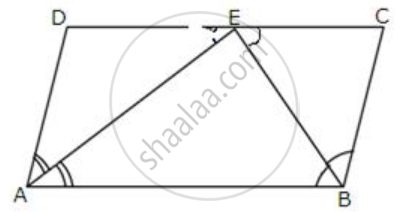
In the given figure, ABCD is a parallelogram.
Prove that: AB = 2 BC.
In parallelogram ABCD, the bisector of angle A meets DC at P and AB = 2 AD.
Prove that:
(i) BP bisects angle B.
(ii) Angle APB = 90o.
PQRS is a parallelogram. T is the mid-point of PQ and ST bisects ∠PSR.
Prove that: QR = QT
In the given figure, MP is the bisector of ∠P and RN is the bisector of ∠R of parallelogram PQRS. Prove that PMRN is a parallelogram.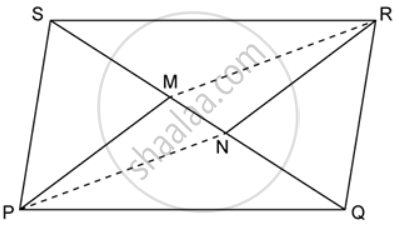
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that: BC = BE.
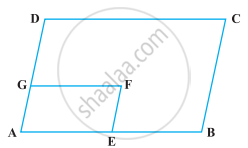
In the following figure, it is given that BDEF and FDCE are parallelograms. Can you say that BD = CD? Why or why not?

In the following figure, ABCD and AEFG are two parallelograms. If ∠C = 55º, determine ∠F.