Advertisements
Advertisements
Question
The area between x = y2 and x = 4 is divided into two equal parts by the line x = a, find the value of a.
Solution
The line, x = a, divides the area bounded by the parabola and x = 4 into two equal parts.
∴ Area OAD = Area ABCD
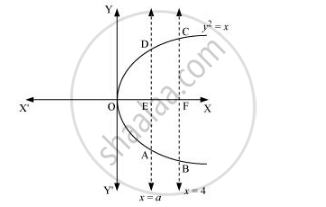
It can be observed that the given area is symmetrical about x-axis.
⇒ Area OED = Area EFCD
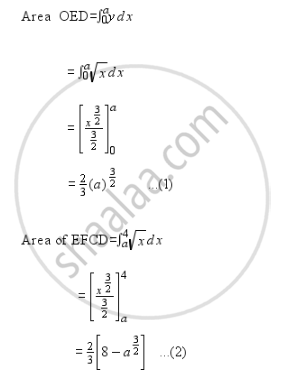
From (1) and (2), we obtain

APPEARS IN
RELATED QUESTIONS
Find the area of the region bounded by the curve y2 = x and the lines x = 1, x = 4 and the x-axis.
Find the area of the region bounded by the curve y2 = 4x and the line x = 3
Find the area enclosed between the parabola y2 = 4ax and the line y = mx
Find the area enclosed between the parabola 4y = 3x2 and the straight line 3x - 2y + 12 = 0.
Find the area bounded by the circle x2 + y2 = 16 and the line `sqrt3 y = x` in the first quadrant, using integration.
Find the area of the region bounded by the parabola y2 = 16x and the line x = 4.
Find the area of the region bounded by the following curve, the X-axis and the given line:
y = 2 – x2, x = –1, x = 1
Find the area of the region bounded by the parabola y2 = 4x and the line x = 3.
Area of the region bounded by x2 = 16y, y = 1 and y = 4 and the Y-axis, lying in the first quadrant is _______.
Fill in the blank :
Area of the region bounded by y = x4, x = 1, x = 5 and the X-axis is _______.
The area of the region bounded by y2 = 4x, the X-axis and the lines x = 1 and x = 4 is _______.
State whether the following is True or False :
The area bounded by the curve x = g (y), Y-axis and bounded between the lines y = c and y = d is given by `int_"c"^"d"x*dy = int_(y = "c")^(y = "d") "g"(y)*dy`
If the curve, under consideration, is below the X-axis, then the area bounded by curve, X-axis and lines x = a, x = b is positive.
Solve the following :
Find the area of the region bounded by the curve y = x2 and the line y = 10.
Area of the region bounded by the curve x = y2, the positive Y axis and the lines y = 1 and y = 3 is ______
State whether the following statement is True or False:
The area of portion lying below the X axis is negative
The area of the shaded region bounded by two curves y = f(x), and y = g(x) and X-axis is `int_"a"^"b" "f"(x) "d"x + int_"a"^"b" "g"(x) "d"x`
The area bounded by the parabola x2 = 9y and the lines y = 4 and y = 9 in the first quadrant is ______
The area of the region bounded by y2 = 25x, x = 1 and x = 2 the X axis is ______
Find the area of the region bounded by the curve y = `sqrt(2x + 3)`, the X axis and the lines x = 0 and x = 2
Find area of the region bounded by the curve y = – 4x, the X-axis and the lines x = – 1 and x = 2
Find area of the region bounded by the parabola x2 = 4y, the Y-axis lying in the first quadrant and the lines y = 3
Find the area of the region bounded by the curve y = `sqrt(36 - x^2)`, the X-axis lying in the first quadrant and the lines x = 0 and x = 6
Find the area of the circle x2 + y2 = 62
Area bounded by the curve xy = 4, X-axis between x = 1, x = 5 is ______.
Area enclosed between the curve y2(4 - x) = x3 and line x = 4 above X-axis is ______.
The area of the region bounded by the curve y = x IxI, X-axis and the ordinates x = 2, x = –2 is ______.
The area included between the parabolas y2 = 4a(x +a) and y2 = 4b(x – a), b > a > 0, is
The area of the circle `x^2 + y^2 = 16`, exterior to the parabola `y = 6x`
The slope of a tangent to the curve y = 3x2 – x + 1 at (1, 3) is ______.
Area in first quadrant bounded by y = 4x2, x = 0, y = 1 and y = 4 is ______.
Area bounded by y = sec2x, x = `π/6`, x = `π/3` and x-axis is ______.
The area (in sq. units) of the region {(x, y) : y2 ≥ 2x and x2 + y2 ≤ 4x, x ≥ 0, y ≥ 0} is ______.
The area bounded by the curve | x | + y = 1 and X-axis is ______.
Find the area of the regions bounded by the line y = −2x, the X-axis and the lines x = −1 and x = 2.
