Advertisements
Advertisements
Question
The length of a rectangular field is thrice of its width. If the perimeter of this field is 1.6km, find its area in sq.m.
Solution
Let the breadth of the rectangle = x m length and of the rectangle = 3x m
The perimeter of a rectangle with length I and breadth b = P = 2(l + b)
∴ The perimeter of a rectangle with length 3x and breadth x = P = 2(3x + x)
= 2(4x) = 8x
⇒ 8x = 1.6km
= 1.6 x 1000m
= 1600m
⇒ x = `(1600)/(8)`
= 200m
⇒ the breadth of the rectangle = 200m length
and of the rectangle = 3(200) = 600m
The area of a rectangle with length l and breadth b = A = l x b
The area of a rectangle with length 600 and breadth 200 = A = 600 x 200 = 120000m2.
APPEARS IN
RELATED QUESTIONS
In the following figures, ABCD is a parallelogram.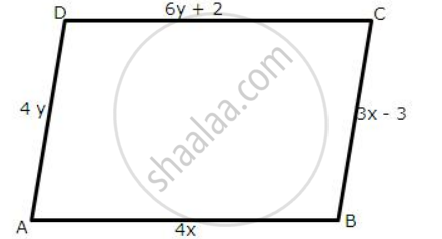
find the values of x and y.
State, 'true' or 'false'
If the diagonals of a quadrilateral bisect each other at right angle, the quadrilateral is a square.
In the given figure ABCD is a rhombus with angle A = 67°

If DEC is an equilateral triangle, calculate:
- ∠CBE
- ∠DBE
The given figure shows a square ABCD and an equilateral triangle ABP. 
Calculate: (i) ∠AOB
(ii) ∠BPC
(iii) ∠PCD
(iv) Reflex ∠APC
Prove that the median of a triangle divides it into two triangles of equal area.
A quadrilateral ABCD is such that diagonals BD divides its area into two equal parts. Prove that BD bisects AC.
Find the area of a parallelogram whose base is 12cm and the height is 5cm.
In a rectangle ABCD, AB = 7 cm and AD = 25 cm. Find the height of a triangle whose base is AB and whose area is two times the area of the rectangle ABCD.
One side of a parallelogram is 12cm and the altitude corresponding to i is 8cm. If the length of the altitude corresponding to its adjacent side is 16cm, find the length of the adjacent side.
The area of a floor of a rectangular room is 360m2. If its length is 24cm, find its perimeter.
A rectangular field 240m long has an area 36000m2. Find the cost of fencing the field at Rs.2.50per m.
A rectangular floor 45 in long and 12 m broad is to be paved exactly with square tiles, of side 60 cm. Find the total number of tiles required to pave it.
If a carpet is laid on the floor such as a space of 50 cm exists between its edges and the edges of the floor, find what fraction of the floor is uncovered.
A rectangular hall of 40m by 24m is covered with carpets of size 6m x 4m. Find the number of carpets required to cover the hall.
Find the area of a square whose diagonal is `12sqrt(12)"cm"`
The perimeter of a square is 128cm and that of another is 96cm. Find the perimeter and the diagonal of a square whose area is equal to the sum of the areas of these two squares.
A rectangular field is 80m long and 50m wide. A 4m wide runs through the centre of the field parallel to the length and breadth of the field. Find the total area of the roads.
A footpath of uniform width runs all around the inside of a rectangular garden of 40 m x 30 m. If the path occupies 136 m2, find the width of the path.
PQRS is a square with each side 6cm. T is a point on QR such that the `"area of ΔPQT"/"area of trapezium PTRS" = (1)/(3)` Find the length of TR.
Give reason for the following :
A square can be thought of as a special rectangle.
Name polygon.

Make two more examples of this.
