Advertisements
Advertisements
प्रश्न
A chord of a circle of radius 12 cm subtends an angle of 120° at the centre. Find the area of the corresponding segment of the circle. [Use π = 3.14 and `sqrt3 = 1.73` ]
उत्तर
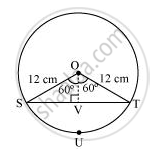
Let us draw a perpendicular OV on chord ST. It will bisect the chord ST.
SV = VT
In ΔOVS,
`(OV)/(OS) = cos 60º`
`(OV)/12 = 1/2`
OV = 6 cm
`(SV)/(SO) = sin 60^@ = sqrt3/2`
`(SV)/12 = sqrt3/2`
`SV = 6sqrt3 cm`
ST = 2SV
= `2xx6sqrt3`
= `12sqrt3 "cm"`
Area of ΔOST =` 1/2 xx ST xx OV`
`1/2xx12sqrt3xx6`
= `36sqrt3`
= 36 × 1.73
= 62.28 cm2
Area of sector OSUT = `120^@/360^@ xx pi(12)^2`
`=1/3xx3.14 xx 144 = 150.72 cm^2`
Area of segment SUT = Area of sector ΔSUT − Area of ΔOST
= 150.72 − 62.28
= 88.44 cm2
APPEARS IN
संबंधित प्रश्न
In Fig. 3, AP and BP are tangents to a circle with centre O, such that AP = 5 cm and ∠APB = 60°. Find the length of chord AB.
Find the area of a quadrant of a circle whose circumference is 22 cm. [Use `pi = 22/7`]
Area of a sector of angle p (in degrees) of a circle with radius R is ______.
The area of sector of circle of radius 5cm is 5𝜋 cm2. Find the angle contained by the sector.
A chord 10 cm long is drawn in a circle whose radius is 5√2 cm. Find the area of both
segments
AB is the diameter of a circle, centre O. C is a point on the circumference such that ∠COB = 𝜃. The area of the minor segment cutoff by AC is equal to twice the area of sector BOC.Prove that `"sin"theta/2. "cos"theta/2= pi (1/2−theta/120^@)`
Area of a sector of central angle 200° of a circle is 770 cm2. Find the length of the corresponding arc of this sector.
The radius of a circle is 17.5 cm. Find the area of the sector enclosed by two radii and an arc 44 cm in length.
The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
Four cows are tethered at the four corners of a square field of side 50 m such that the each can graze the maximum unshared area. What area will be left ungrazed?
A chord of length 6 cm is at a distance of 7.2 cm from the centre of a circle. Another chord of the same circle is of length 14.4 cm. Find its distance from the centre.
Find the area of the sector whose arc length and radius are 8 cm and 3 cm respectively.
The diameter of a sphere is 6 cm, Find the total surface area of the sphere. (π = 3.14)
If the area of a circle is numerically equal to twice its circumference, then the diameter of the circle is ____________.
Find the area of the shaded region where ABC is a quadrant of radius 5cm and a semicircle is drawn with BC as diameter.

The circumference of a circle is 100 cm. The side of a square inscribed in the circle is ______.
Find the area of the minor segment of a circle of radius 14 cm, when the angle of the corresponding sector is 60°.
In figure, arcs have been drawn with radii 14 cm each and with centres P, Q and R. Find the area of the shaded region.
Find the area of the sector of a circle of radius 7 cm and of central angle 90°. Also, find the area of corresponding major sector.
With vertices A, B and C of ΔABC as centres, arcs are drawn with radii 14 cm and the three portions of the triangle so obtained are removed. Find the total area removed from the triangle.
