Advertisements
Advertisements
प्रश्न
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cos2 C + cosec2 C
उत्तर
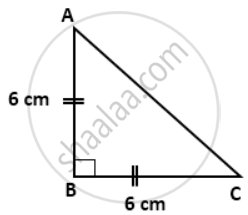
ΔABC is an isosceles right-angled triangle.
∴ AC2
= AB2 + BC2
= 62 + 62
= 36 + 36
= 72
⇒ AC = `6sqrt(2)"cm"`
cos2 C + cosec2 C
= `(1/sqrt(2))^2 + (sqrt(2))^2`
= `(1)/(2) + 2`
= `(5)/(2)`.
APPEARS IN
संबंधित प्रश्न
If `sin A = 9/41` compute cos 𝐴 𝑎𝑛𝑑 tan 𝐴
If A and B are acute angles such that tan A = 1/2, tan B = 1/3 and tan (A + B) = `(tan A + tan B)/(1- tan A tan B)` A + B = ?
If cos θ = `3/5` , show that `((sin theta - cot theta ))/(2tan theta)=3/160`
If x = cot A + cos A and y = cot A – cos A then prove that `((x-y)/(x+y))^2 + ((x-y)/2)^2=1`
If A = 600 and B = 300, verify that:
cos (A + B) = cos A cos B - sin A sin B
cos 40° = sin ______°
From the following figure, find the values of :
(i) sin A
(ii) sec A
(iii) cos2 A + sin2A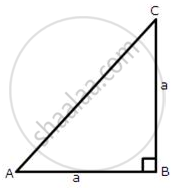
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cos C
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`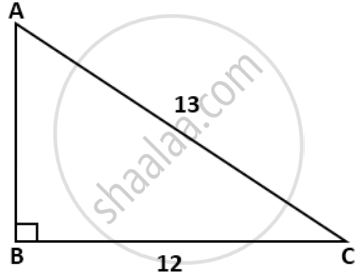
If sin θ = `"a"/sqrt("a"^2 + "b"^2)`, then show that b sin θ = a cos θ
