Advertisements
Advertisements
प्रश्न
In the adjacent figure, `square`ABCD is a trapezium AB || DC. Points M and N are midpoints of diagonal AC and DB respectively then prove that MN || AB.

उत्तर
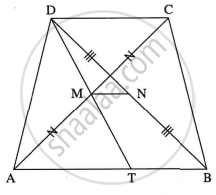
Given: `square`ABCD is a trapezium. AB || DC
Points M and N are the midpoints of diagonals AC and DB respectively.
To prove: MN || AB
Construction: Draw line DM which intersects side AB at point T.
Proof:
side DC || side AB …(Given)
And seg AC is a transversal line.
∴ ∠DAC ≅ ∠BAC ...(alternate angles)
∴ ∠DCM ≅ ∠TAM ...(i) ...(A-M-C and A-T-B)
In ∆DCM and ∆TAM,
∠DCM ≅ ∠TAM ...[From (i)]
seg MC ≅ seg MA ...(Point M is the midpoint of seg AC.)
∠DCM ≅ ∠TAM ...(Vertically opposite angles)
∴ ∆DCM ≅ ∆TAM ...(ASA test)
seg DM ≅ seg MT ...(c.s.c.t) ...(ii)
In ∆DTB,
Point N is the midpoint of line DB. ...(Given)
Point M is the midpoint of line DT. ...[From (ii)]
∴ seg MN || side TB ...(Midpoint Theorem)
∴ seg MN || seg AB ...(A-T-B)
APPEARS IN
संबंधित प्रश्न
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
In trapezium ABCD, sides AB and DC are parallel to each other. E is mid-point of AD and F is mid-point of BC.
Prove that: AB + DC = 2EF.
In parallelogram PQRS, L is mid-point of side SR and SN is drawn parallel to LQ which meets RQ produced at N and cuts side PQ at M. Prove that M is the mid-point of PQ.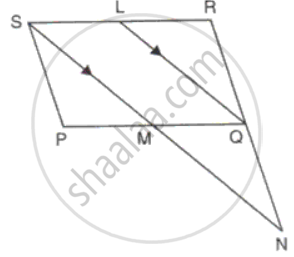
In ΔABC, BE and CF are medians. P is a point on BE produced such that BE = EP and Q is a point on CF produced such that CF = FQ. Prove that: QAP is a straight line.
Prove that the straight lines joining the mid-points of the opposite sides of a quadrilateral bisect each other.
In the given figure, ABCD is a trapezium. P and Q are the midpoints of non-parallel side AD and BC respectively. Find: PQ, if AB = 12 cm and DC = 10 cm.
In ΔABC, the medians BE and CD are produced to the points P and Q respectively such that BE = EP and CD = DQ. Prove that: Q A and P are collinear.
In the given figure, PS = 3RS. M is the midpoint of QR. If TR || MN || QP, then prove that: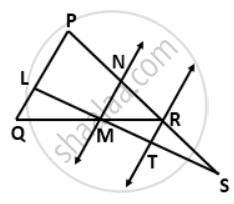
ST = `(1)/(3)"LS"`
The figure formed by joining the mid-points of the sides of a quadrilateral ABCD, taken in order, is a square only if, ______.
P, Q, R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.
