Advertisements
Advertisements
प्रश्न
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
उत्तर १
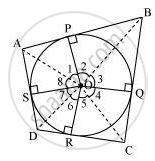
Let ABCD be a quadrilateral circumscribing a circle centred at O such that it touches the circle at point P, Q, R, and S. Let us join the vertices of the quadrilateral ABCD to the centre of the circle.
Consider ΔOAP and ΔOAS,
AP = AS .....(Tangents from the same point)
OP = OS ..... (Radii of the same circle)
OA = OA ....(Common side)
ΔOAP ≅ ΔOAS .... (SSS congruence criterion)
Therefore, A ↔ A, P ↔ S, O ↔ O
And thus, ∠POA = ∠AOS
∠1 = ∠8
Similarly,
∠2 = ∠3
∠4 = ∠5
∠6 = ∠7
∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 + ∠7 + ∠8 = 360º
(∠1 + ∠8) + (∠2 + ∠3) + (∠4 + ∠5) + (∠6 + ∠7) = 360º
2∠1 + 2∠2 + 2∠5 + 2∠6 = 360º
2(∠1 + ∠2) + 2(∠5 + ∠6) = 360º
(∠1 + ∠2) + (∠5 + ∠6) = 180º
∠AOB + ∠COD = 180º
Similarly, we can prove that ∠BOC + ∠DOA = 180º
Hence, opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
उत्तर २
Let ABCD be a quadrilateral circumscribing a circle with centre at O.
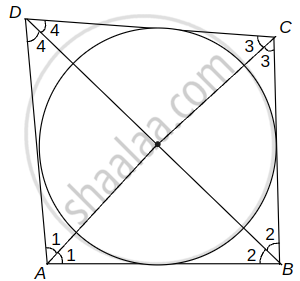
Join OA, OB, OC and OD.
Here, Let ∠DAO = ∠BAO = ∠1 ...[∵ AB and AD are tangents]
Similarly, ∠ABO = ∠CBO = ∠2 ...[∵ BA and BC are tangents]
And Let ∠BCO = ∠DCO = ∠3 ...[∵ CB and CD are tangents]
And Let ∠CDO = ∠ADO = ∠4 ...[∵ DC and DA are tangents]
Sum of all angles at the centre is 360°.
Also, the sum of the angles in quadrilateral, ABCD = 360°
∴ 2(∠1 + ∠2 + ∠3 + ∠4) = 360°
⇒ ∠1 + ∠2 + ∠3 + ∠4 = 180° ...(i)
Now, In ΔAOB,
∠AOB = 180° – (∠1 + ∠2) ...(ii)
In ΔCOD, ∠COD = 180° – (∠3 + ∠4) ...(iii)
On adding equations (ii) and (iii), we get
∠AOB + ∠COD = 360° – (∠1 + ∠2 + ∠3 + ∠4)
= 360° – 180°
= 180° ... [From equation (i)]
Since AB and CD subtend supplementary angles at O.
Thus, opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
APPEARS IN
संबंधित प्रश्न
Prove that the lengths of the tangents drawn from an external point to a circle are equal.
From an external point P, tangents PA and PB are drawn to a circle with centre O. If ∠PAB = 50°, then find ∠AOB.
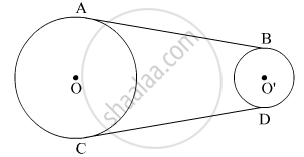
In the figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB = CD.
Prove that the tangents drawn at the end points of a chord of a circle make equal angles with the chord.
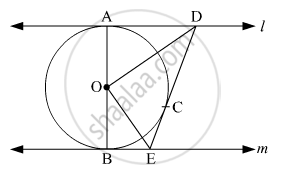
In fig. 6, l and m are two parallel tangents to a circle with centre O, touching the circle at A and B respectively. Another tangent at C intersects the line l at D and m at E. Prove that ∠DOE = 90° ?
Prove that the centre of a circle touching two intersecting lines lies on the angle bisector of the lines.
In the given circle with center o, ∠ABC=100°, ∠ACD=40° and CT is tangent to the circle at C. find ∠ADC and ∠DCT.

In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.
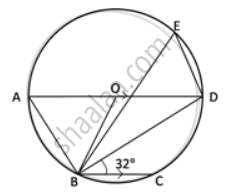
Find: ∠BED
In the given figure PA = 6, PB = 4 and PC = 8. Find PD
Prove that the lengths of two tangent segments drawn to the circle from an external point are equal.
In Fig. 4, a circle is inscribed in a ΔABC having sides BC = 8 cm, AB = 10 cm and AC = 12 cm. Find the lengths BL, CM and AN.

The length of tangents drawn from an external point to the circle ______
Two concentric circles of radii a and b (a > b) are given. Find the length of the chord of the larger circle which touches the smaller circle.
In a circle of radius 5 cm, AB and AC are the two chords such that AB = AC = 6 cm. Find the length of the chord BC.
In a circle of radius 17 cm, two parallel chords are drawn on opposite sides of a diameter. The distance between the chords is 23 cm. If the length of one chord is 16 cm, then the length of the other is ______
In fig, O is the centre of the circle, CA is tangent at A and CB is tangent at B drawn to the circle. If ∠ACB = 75°, then ∠AOB = ______

The angle between two tangents to a circle may be 0°.
From a point P, two tangents PA and PB are drawn to a circle C(0, r). If OP = 2r, then find ∠APB. What type of triangle is APB?

In the given figure, perimeter of ΔPQR is 20 cm. Find the length of tangent PA.

PA and PB are tangents drawn to a circle of centre O from an external point P. Chord AB makes an angle of 30° with the radius at the point of contact. If length of the chord is 6 cm, find the length of the tangent PA and the length of the radius OA.