Advertisements
Advertisements
प्रश्न
ΔRST ~ ΔUAY, In ΔRST, RS = 6 cm, ∠S = 50°, ST = 7.5 cm. The corresponding sides of ΔRST and ΔUAY are in the ratio 5 : 4. Construct ΔUAY.
उत्तर
Given that ΔRST ~ ΔUAY.
In ΔRST, RS = 6 cm, m∠S = 50°, ST = 7.5 cm.
Given that the corresponding sides of ΔRST and ΔUAY are in the ratio 5 : 4.
`therefore "RS"/"UA"="ST"/"AY"="RT"/"UY"=5/4;`
∠S =∠A = 50º
`therefore"RS"/"UA"=5/4`
`therefore6/"UA"=5/4`
`therefore(6xx4)/5="UA"`
`therefore "UA"=4.8 "cm"`
Similarly,
`"ST"/"AY"=5/4;`
`therefore7.5/"AY"=5/4`
`therefore(7.5xx4)/5="AY"`
`therefore"AY"=6" cm"`
Therefore, In ΔUAY, UA = 4.8 cm, AY = 6 cm and m∠A = 50°

APPEARS IN
संबंधित प्रश्न
Find the ratio in which the line segment joining the points A(3,- 3) and B(- 2, 7) is divided by x-axis. Also find the coordinates of the point of division.
Construct a triangle ABC with BC = 7 cm, ∠B = 60° and AB = 6 cm. Construct another triangle whose sides are `3/4` times the corresponding sides of ∆ABC.
Construct a triangle of sides 4 cm, 5cm and 6cm and then a triangle similar to it whose sides are `2/3` of the corresponding sides of the first triangle. Give the justification of the construction.
Construct a triangle with sides 5 cm, 6 cm and 7 cm and then another triangle whose sides are `7/5` of the corresponding sides of the first triangle. Give the justification of the construction.
Construct an isosceles triangle whose base is 8 cm and altitude 4 cm and then another triangle whose side are `1 1/2` times the corresponding sides of the isosceles triangle.
Give the justification of the construction
Draw a triangle ABC with side BC = 7 cm, ∠B = 45°, ∠A = 105°. Then, construct a triangle whose sides are `4/3 `times the corresponding side of ΔABC. Give the justification of the construction.
Construct an isosceles triangle with base 8 cm and altitude 4 cm. Construct another triangle whose sides are `2/3` times the corresponding sides of the isosceles triangle.
Draw a line segment of length 7 cm and divide it internally in the ratio 2 : 3.
Draw a right triangle in which the sides (other than the hypotenuse) are of lengths 4 cm and 3 cm. Now construct another triangle whose sides are `3/5` times the corresponding sides of the given triangle.
Determine a point which divides a line segment of length 12 cm internally in the ratio 2 : 3 Also, justify your construction.
Construct a triangle similar to a given ΔABC such that each of its sides is (2/3)rd of the corresponding sides of ΔABC. It is given that BC = 6 cm, ∠B = 50° and ∠C = 60°.
Construct the circumcircle and incircle of an equilateral ∆XYZ with side 6.5 cm and centre O. Find the ratio of the radii of incircle and circumcircle.
Draw a ∆ABC in which AB = 4 cm, BC = 5 cm and AC = 6 cm. Then construct another triangle whose sides are\[\frac{3}{5}\] of the corresponding sides of ∆ABC ?
∆AMT ~ ∆AHE. In ∆AMT, AM = 6.3 cm, ∠TAM = 50°, AT = 5.6 cm. `"AM"/"AH" = 7/5`. Construct ∆AHE.
∆ABC ~ ∆LBN. In ∆ABC, AB = 5.1 cm, ∠B = 40°, BC = 4.8 cm, \[\frac{AC}{LN} = \frac{4}{7}\]. Construct ∆ABC and ∆LBN.
Find the ratio in which point T(–1, 6)divides the line segment joining the points P(–3, 10) and Q(6, –8).
Find the co-ordinates of the points of trisection of the line segment AB with A(2, 7) and B(–4, –8).
If A (20, 10), B(0, 20) are given, find the coordinates of the points which divide segment AB into five congruent parts.
Draw a line segment AB of length 7 cm. Using ruler and compasses, find a point P on AB such that `(AP)/(AB)=3/5`.
Draw a right triangle in which the sides (other than the hypotenuse) are of lengths 4 cm and 3 cm. Now construct another triangle whose sides are \[\frac{3}{5}\] times the corresponding sides of the given triangle.
Find the ratio in which the segment joining the points (1, –3) and (4, 5) is divided by the x-axis? Also, find the coordinates of this point on the x-axis.
Choose the correct alternative:
______ number of tangents can be drawn to a circle from the point on the circle.
ΔPQR ~ ΔABC. In ΔPQR, PQ = 3.6cm, QR = 4 cm, PR = 4.2 cm. Ratio of the corresponding sides of triangle is 3 : 4, then construct ΔPQR and ΔABC
ΔABC ~ ΔPBR, BC = 8 cm, AC = 10 cm , ∠B = 90°, `"BC"/"BR" = 5/4` then construct ∆ABC and ΔPBR
By geometrical construction, it is possible to divide a line segment in the ratio ______.
Draw the line segment AB = 5cm. From the point A draw a line segment AD = 6cm making an angle of 60° with AB. Draw a perpendicular bisector of AD. Select the correct figure.
If I ask you to construct ΔPQR ~ ΔABC exactly (when we say exactly, we mean the exact relative positions of the triangles) as given in the figure, (Assuming I give you the dimensions of ΔABC and the Scale Factor for ΔPQR) what additional information would you ask for?
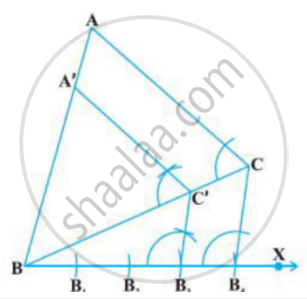
The image of construction of A’C’B a similar triangle of ΔACB is given below. Then choose the correct option.
Construction of similar polygons is similar to that of construction of similar triangles. If you are asked to construct a parallelogram similar to a given parallelogram with a given scale factor, which of the given steps will help you construct a similar parallelogram?
A point C divides a line segment AB in the ratio 5 : 6. The ratio of lengths AB: BC is ______.
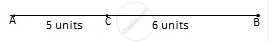
The point W divides the line XY in the ratio m : n. Then, the ratio of lengths of the line segments XY : WX is ______.
To divide a line segment, the ratio of division must be ______.
Draw a line segment of length 7 cm. Find a point P on it which divides it in the ratio 3:5.
Two line segments AB and AC include an angle of 60° where AB = 5 cm and AC = 7 cm. Locate points P and Q on AB and AC, respectively such that AP = `3/4` AB and AQ = `1/4` AC. Join P and Q and measure the length PQ.
Draw a parallelogram ABCD in which BC = 5 cm, AB = 3 cm and ∠ABC = 60°, divide it into triangles BCD and ABD by the diagonal BD. Construct the triangle BD' C' similar to ∆BDC with scale factor `4/3`. Draw the line segment D'A' parallel to DA where A' lies on extended side BA. Is A'BC'D' a parallelogram?
Draw a line segment of length 7 cm and divide it in the ratio 5 : 3.
