Advertisements
Advertisements
प्रश्न
Find the area of the minor segment of the circle x2 + y2 = 4 cut off by the line x = 1, using integration.
उत्तर
Given equation of circle x2 + y2 = 4
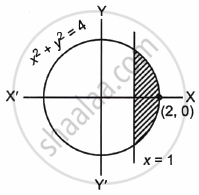
Required Area = `int_1^2 y dx`
= `2int_1^2 sqrt(4 - x^2)dx`
= `2[x/2 sqrt(4 - x^2) + 4/2 sin^-1 x/2]_1^2`
= `2[0 + 2 sin^-1 (1) - (1/2 sqrt(3) + 2 sin^-1 (1/2))]`
= `4(π/2) - sqrt(3) - 4(π/6)`
= `(2π - (2π)/3) - sqrt(3)`
= `(4π - 3sqrt(3))/3`
APPEARS IN
संबंधित प्रश्न
Find the area of the region bounded by the parabola y2 = 4ax and the line x = a.
Draw a rough sketch of the curve \[y = \frac{x}{\pi} + 2 \sin^2 x\] and find the area between the x-axis, the curve and the ordinates x = 0 and x = π.
Find the area bounded by the ellipse \[\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1\] and the ordinates x = ae and x = 0, where b2 = a2 (1 − e2) and e < 1.
Find the area of the region included between the parabola y2 = x and the line x + y = 2.
Find the area, lying above x-axis and included between the circle x2 + y2 = 8x and the parabola y2 = 4x.
Find the area of the region bounded by the curves y = x − 1 and (y − 1)2 = 4 (x + 1).
Find the area bounded by the curves x = y2 and x = 3 − 2y2.
In what ratio does the x-axis divide the area of the region bounded by the parabolas y = 4x − x2 and y = x2− x?
The area included between the parabolas y2 = 4x and x2 = 4y is (in square units)
The closed area made by the parabola y = 2x2 and y = x2 + 4 is __________ .
Area bounded by parabola y2 = x and straight line 2y = x is _________ .
The area bounded by the curve y = 4x − x2 and the x-axis is __________ .
The area bounded by the curve y2 = 8x and x2 = 8y is ___________ .
Find the area of the region bound by the curves y = 6x – x2 and y = x2 – 2x
Find the area of the curve y = sin x between 0 and π.
The area enclosed by the circle x2 + y2 = 2 is equal to ______.
Using integration, find the area of the region bounded by the line 2y = 5x + 7, x- axis and the lines x = 2 and x = 8.
Compute the area bounded by the lines x + 2y = 2, y – x = 1 and 2x + y = 7.
The area of the region bounded by the curve x2 = 4y and the straight line x = 4y – 2 is ______.
Using integration, find the area of the region in the first quadrant enclosed by the line x + y = 2, the parabola y2 = x and the x-axis.
Area of the region bounded by the curve `y^2 = 4x`, `y`-axis and the line `y` = 3 is:
What is the area of the region bounded by the curve `y^2 = 4x` and the line `x` = 3.
Using integration, find the area of the region bounded by the curves x2 + y2 = 4, x = `sqrt(3)`y and x-axis lying in the first quadrant.
Let a and b respectively be the points of local maximum and local minimum of the function f(x) = 2x3 – 3x2 – 12x. If A is the total area of the region bounded by y = f(x), the x-axis and the lines x = a and x = b, then 4A is equal to ______.
Let P(x) be a real polynomial of degree 3 which vanishes at x = –3. Let P(x) have local minima at x = 1, local maxima at x = –1 and `int_-1^1 P(x)dx` = 18, then the sum of all the coefficients of the polynomial P(x) is equal to ______.
Using integration, find the area of the region bounded by y = mx (m > 0), x = 1, x = 2 and the X-axis.
Using integration, find the area bounded by the curve y2 = 4ax and the line x = a.
Using integration, find the area of the region bounded by the curve y2 = 4x and x2 = 4y.
