Advertisements
Advertisements
प्रश्न
Using integration, find the area of the region bounded by the curve y2 = 4x and x2 = 4y.
उत्तर
Given that the curves are y2 = 4x and x2 = 4y.
Now, the graph of the provided curves is as follows:
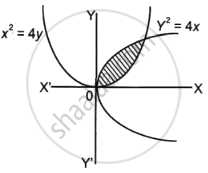
The given equations are:
y2 = 4x ...(i)
And x2 = 4y
`y = x^2/4` ...(ii)
Put the value of (ii) in (i), we get
`(x^2/4)^2 = 4x`
`\implies x^4/16 = 4x`
`\implies` x4 = 4 × 16x
`\implies` x4 – 64x = 0
`\implies` x(x3 – 64) = 0
`\implies` x = 0 or x = 4
The curve is rewritten as follows:
y2 = 4x
`\implies y = sqrt(4x) = 2sqrt(x)`
`\implies` x2 = 4y
`\implies y = x^2/4`
Now, the area of the bounded region is given as:
A = `int_0^4 (2sqrt(x) - x^2/4)dx`
= `[2 xx x^(3/2)/(3/2) - x^3/12]_0^4`
= `[(4/3 xx 4^(3//2)) - 4^3/12] - 0`
= `[(4 xx 8)/3 - 64/12]`
= `(128 - 64)/12`
= `64/12`
= `16/3` sq.units
APPEARS IN
संबंधित प्रश्न
Draw a rough sketch of the curve and find the area of the region bounded by curve y2 = 8x and the line x =2.
Find the area lying above the x-axis and under the parabola y = 4x − x2.
Sketch the region {(x, y) : 9x2 + 4y2 = 36} and find the area of the region enclosed by it, using integration.
Using integration, find the area of the region bounded by the following curves, after making a rough sketch: y = 1 + | x + 1 |, x = −2, x = 3, y = 0.
Draw a rough sketch of the curve \[y = \frac{x}{\pi} + 2 \sin^2 x\] and find the area between the x-axis, the curve and the ordinates x = 0 and x = π.
Find the area of the region included between the parabola y2 = x and the line x + y = 2.
Find the area of the region bounded by \[y = \sqrt{x}\] and y = x.
Find the area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2= 32.
Find the area bounded by the lines y = 4x + 5, y = 5 − x and 4y = x + 5.
Find the area of the region enclosed between the two curves x2 + y2 = 9 and (x − 3)2 + y2 = 9.
The area of the region bounded by the parabola (y − 2)2 = x − 1, the tangent to it at the point with the ordinate 3 and the x-axis is _________ .
The closed area made by the parabola y = 2x2 and y = x2 + 4 is __________ .
The area of the region bounded by the parabola y = x2 + 1 and the straight line x + y = 3 is given by
The area bounded by the curve y = f (x), x-axis, and the ordinates x = 1 and x = b is (b −1) sin (3b + 4). Then, f (x) is __________ .
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using vertical strips.
Find the equation of the parabola with latus-rectum joining points (4, 6) and (4, -2).
Using integration, find the area of the smaller region bounded by the ellipse `"x"^2/9+"y"^2/4=1`and the line `"x"/3+"y"/2=1.`
The area enclosed by the circle x2 + y2 = 2 is equal to ______.
Find the area of the region bounded by the parabola y2 = 2px, x2 = 2py
Find the area of the region bounded by y = `sqrt(x)` and y = x.
Find the area enclosed by the curve y = –x2 and the straight lilne x + y + 2 = 0
Find the area of the region bounded by the curve y2 = 2x and x2 + y2 = 4x.
Area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2 = 32 is ______.
Using integration, find the area of the region in the first quadrant enclosed by the line x + y = 2, the parabola y2 = x and the x-axis.
The area of the region bounded by the line y = 4 and the curve y = x2 is ______.
Area of the region bounded by the curve y = |x + 1| + 1, x = –3, x = 3 and y = 0 is
The area bounded by `y`-axis, `y = cosx` and `y = sinx, 0 ≤ x - (<pi)/2` is
Let f(x) be a non-negative continuous function such that the area bounded by the curve y = f(x), x-axis and the ordinates x = `π/4` and x = `β > π/4` is `(βsinβ + π/4 cos β + sqrt(2)β)`. Then `f(π/2)` is ______.
Let a and b respectively be the points of local maximum and local minimum of the function f(x) = 2x3 – 3x2 – 12x. If A is the total area of the region bounded by y = f(x), the x-axis and the lines x = a and x = b, then 4A is equal to ______.
