Advertisements
Advertisements
प्रश्न
Using integration, find the area of the smaller region bounded by the ellipse `"x"^2/9+"y"^2/4=1`and the line `"x"/3+"y"/2=1.`
उत्तर
Given the equation of the ellipse is `"x"^2/9+"y"^2/4=1`
Let `"y"_1=2/3sqrt(9-"x"^2)` and
equation of the line is `"x"/3+"y"/2=1`
Let `"y"_2=2/3(3-"x")`
we have (3, 0) and (0, 2) as the points of intersection of ellipse and line.
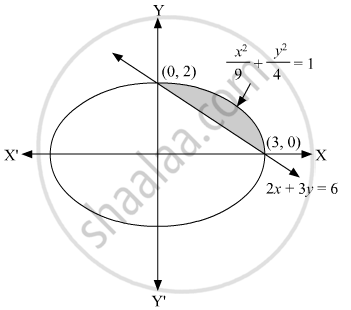
Therefore, the area of a smaller region, A `=int_0^3("y"_1-"y"_2)"dx"`
`"A" =int_0^3[2/3sqrt(9-"x"^2)-2/3(3-"x")]"dx"`
`=int_0^3(2/3sqrt(9-"x"^2))"dx"-int_0^3[2/3(3-"x")]"dx"`
`=2/3["x"/2sqrt(9-"x"^2)+9/2sin^-1("x"/3)]_0^3-2/3(3"x"-"x"^2/2)_0^3`
`=2/3[(0+9/2xxpi/2)-0]-2/3(9-9/2-0)`
`=((3pi)/2-3)` sq. unit
APPEARS IN
संबंधित प्रश्न
Prove that the curves y2 = 4x and x2 = 4y divide the area of square bounded by x = 0, x = 4, y = 4 and y = 0 into three equal parts.
Using integration, find the area of the region bounded between the line x = 2 and the parabola y2 = 8x.
Find the area of the region bounded by the parabola y2 = 4ax and the line x = a.
Draw a rough sketch to indicate the region bounded between the curve y2 = 4x and the line x = 3. Also, find the area of this region.
Make a rough sketch of the graph of the function y = 4 − x2, 0 ≤ x ≤ 2 and determine the area enclosed by the curve, the x-axis and the lines x = 0 and x = 2.
Sketch the graph of y = \[\sqrt{x + 1}\] in [0, 4] and determine the area of the region enclosed by the curve, the x-axis and the lines x = 0, x = 4.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Using integration, find the area of the region bounded by the following curves, after making a rough sketch: y = 1 + | x + 1 |, x = −2, x = 3, y = 0.
Sketch the graph y = | x + 3 |. Evaluate \[\int\limits_{- 6}^0 \left| x + 3 \right| dx\]. What does this integral represent on the graph?
Find the area bounded by the curve y = cos x, x-axis and the ordinates x = 0 and x = 2π.
Find the area, lying above x-axis and included between the circle x2 + y2 = 8x and the parabola y2 = 4x.
Find the area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2= 32.
Make a sketch of the region {(x, y) : 0 ≤ y ≤ x2 + 3; 0 ≤ y ≤ 2x + 3; 0 ≤ x ≤ 3} and find its area using integration.
Find the area enclosed by the parabolas y = 4x − x2 and y = x2 − x.
The area of the region bounded by the parabola y = x2 + 1 and the straight line x + y = 3 is given by
Find the coordinates of a point of the parabola y = x2 + 7x + 2 which is closest to the straight line y = 3x − 3.
Sketch the graphs of the curves y2 = x and y2 = 4 – 3x and find the area enclosed between them.
The area enclosed by the circle x2 + y2 = 2 is equal to ______.
Find the area bounded by the curve y = `sqrt(x)`, x = 2y + 3 in the first quadrant and x-axis.
The area of the region bounded by the circle x2 + y2 = 1 is ______.
The area of the region bounded by the curve y = x + 1 and the lines x = 2 and x = 3 is ______.
Using integration, find the area of the region bounded between the line x = 4 and the parabola y2 = 16x.
Using integration, find the area of the region in the first quadrant enclosed by the line x + y = 2, the parabola y2 = x and the x-axis.
Find the area of the region bounded by `y^2 = 9x, x = 2, x = 4` and the `x`-axis in the first quadrant.
Area of figure bounded by straight lines x = 0, x = 2 and the curves y = 2x, y = 2x – x2 is ______.
Let f(x) be a non-negative continuous function such that the area bounded by the curve y = f(x), x-axis and the ordinates x = `π/4` and x = `β > π/4` is `(βsinβ + π/4 cos β + sqrt(2)β)`. Then `f(π/2)` is ______.
Sketch the region bounded by the lines 2x + y = 8, y = 2, y = 4 and the Y-axis. Hence, obtain its area using integration.
Using integration, find the area of the region bounded by y = mx (m > 0), x = 1, x = 2 and the X-axis.
