Advertisements
Advertisements
प्रश्न
Draw a rough sketch of the curve and find the area of the region bounded by curve y2 = 8x and the line x =2.
उत्तर
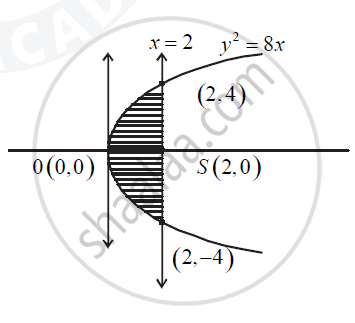
Given equation is y2 = 8x
Comparing with y2 -4ax
we get 4a = 8
i.e a = 2
Given `y^2 = 4(2)x`
`y^2 = 8x``
`:. y = sqrt(8x)`
Also x = 2 meets `y^2 = 8x`
`:. y^2 = 16`
`:. y = +-4`
:. (2,4) and (2,-4) are their point of intersection
:. Required are `A = 2int_0^2 sqrt(8x) dx`
`=2sqrt8 int_0^2 x^("1/2") dx`
`= 4sqrt2 = [x^("3/2")/("3/2")]_0^2`
`= (8sqrt2)/3 [2^"3/2" - 0]`
`= (8sqrt2)/3 xx sqrt8`
`= (8sqrt2xx2sqrt2)/3 = 32/3` sq.units
APPEARS IN
संबंधित प्रश्न
Using integration, find the area bounded by the curve x2 = 4y and the line x = 4y − 2.
Find the area of the region common to the circle x2 + y2 =9 and the parabola y2 =8x
Find the area of the sector of a circle bounded by the circle x2 + y2 = 16 and the line y = x in the ftrst quadrant.
Find the equation of a curve passing through the point (0, 2), given that the sum of the coordinates of any point on the curve exceeds the slope of the tangent to the curve at that point by 5
Find the area lying above the x-axis and under the parabola y = 4x − x2.
Draw a rough sketch of the graph of the curve \[\frac{x^2}{4} + \frac{y^2}{9} = 1\] and evaluate the area of the region under the curve and above the x-axis.
Determine the area under the curve y = \[\sqrt{a^2 - x^2}\] included between the lines x = 0 and x = a.
Show that the areas under the curves y = sin x and y = sin 2x between x = 0 and x =\[\frac{\pi}{3}\] are in the ratio 2 : 3.
Find the area of the region in the first quadrant enclosed by x-axis, the line y = \[\sqrt{3}x\] and the circle x2 + y2 = 16.
Find the area bounded by the parabola y = 2 − x2 and the straight line y + x = 0.
Find the area bounded by the curves x = y2 and x = 3 − 2y2.
Using integration, find the area of the following region: \[\left\{ \left( x, y \right) : \frac{x^2}{9} + \frac{y^2}{4} \leq 1 \leq \frac{x}{3} + \frac{y}{2} \right\}\]
Find the area enclosed by the curves 3x2 + 5y = 32 and y = | x − 2 |.
If the area enclosed by the parabolas y2 = 16ax and x2 = 16ay, a > 0 is \[\frac{1024}{3}\] square units, find the value of a.
The area bounded by the parabola x = 4 − y2 and y-axis, in square units, is ____________ .
If An be the area bounded by the curve y = (tan x)n and the lines x = 0, y = 0 and x = π/4, then for x > 2
The area bounded by the curve y = x4 − 2x3 + x2 + 3 with x-axis and ordinates corresponding to the minima of y is _________ .
The area bounded by the parabola y2 = 4ax, latusrectum and x-axis is ___________ .
Draw a rough sketch of the curve y2 = 4x and find the area of region enclosed by the curve and the line y = x.
The area of the region bounded by the curve x = y2, y-axis and the line y = 3 and y = 4 is ______.
The area of the region bounded by the curve y = x2 + x, x-axis and the line x = 2 and x = 5 is equal to ______.
Find the area of the region bounded by the curve y2 = 2x and x2 + y2 = 4x.
The area of the region bounded by the curve y = sinx between the ordinates x = 0, x = `pi/2` and the x-axis is ______.
The area of the region bounded by the line y = 4 and the curve y = x2 is ______.
If a and c are positive real numbers and the ellipse `x^2/(4c^2) + y^2/c^2` = 1 has four distinct points in common with the circle `x^2 + y^2 = 9a^2`, then
Make a rough sketch of the region {(x, y): 0 ≤ y ≤ x2, 0 ≤ y ≤ x, 0 ≤ x ≤ 2} and find the area of the region using integration.
For real number a, b (a > b > 0),
let Area `{(x, y): x^2 + y^2 ≤ a^2 and x^2/a^2 + y^2/b^2 ≥ 1}` = 30π
Area `{(x, y): x^2 + y^2 ≥ b^2 and x^2/a^2 + y^2/b^2 ≤ 1}` = 18π.
Then the value of (a – b)2 is equal to ______.
Let f(x) be a non-negative continuous function such that the area bounded by the curve y = f(x), x-axis and the ordinates x = `π/4` and x = `β > π/4` is `(βsinβ + π/4 cos β + sqrt(2)β)`. Then `f(π/2)` is ______.
Find the area of the region bounded by the curve x2 = 4y and the line x = 4y – 2.
