Advertisements
Advertisements
Question
Draw a rough sketch of the curve and find the area of the region bounded by curve y2 = 8x and the line x =2.
Solution
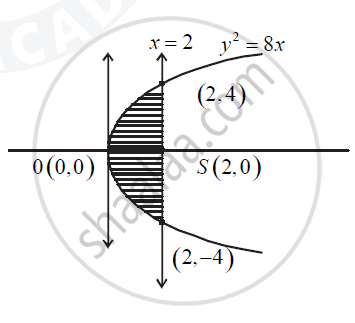
Given equation is y2 = 8x
Comparing with y2 -4ax
we get 4a = 8
i.e a = 2
Given `y^2 = 4(2)x`
`y^2 = 8x``
`:. y = sqrt(8x)`
Also x = 2 meets `y^2 = 8x`
`:. y^2 = 16`
`:. y = +-4`
:. (2,4) and (2,-4) are their point of intersection
:. Required are `A = 2int_0^2 sqrt(8x) dx`
`=2sqrt8 int_0^2 x^("1/2") dx`
`= 4sqrt2 = [x^("3/2")/("3/2")]_0^2`
`= (8sqrt2)/3 [2^"3/2" - 0]`
`= (8sqrt2)/3 xx sqrt8`
`= (8sqrt2xx2sqrt2)/3 = 32/3` sq.units
APPEARS IN
RELATED QUESTIONS
Using integration, find the area of the region bounded by the lines y = 2 + x, y = 2 – x and x = 2.
Find the area bounded by the curve y = sin x between x = 0 and x = 2π.
The area bounded by the curve y = x | x|, x-axis and the ordinates x = –1 and x = 1 is given by ______.
[Hint: y = x2 if x > 0 and y = –x2 if x < 0]
Using integration, find the area of the region bounded between the line x = 2 and the parabola y2 = 8x.
Draw the rough sketch of y2 + 1 = x, x ≤ 2. Find the area enclosed by the curve and the line x = 2.
Find the area of the region {(x, y) : y2 ≤ 8x, x2 + y2 ≤ 9}.
Find the area of the region common to the circle x2 + y2 = 16 and the parabola y2 = 6x.
Find the area of the region included between the parabola y2 = x and the line x + y = 2.
Find the area of the region bounded by \[y = \sqrt{x}, x = 2y + 3\] in the first quadrant and x-axis.
Find the area bounded by the parabola y = 2 − x2 and the straight line y + x = 0.
Find the area of the region between the parabola x = 4y − y2 and the line x = 2y − 3.
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using horizontal strips.
The area bounded by y = 2 − x2 and x + y = 0 is _________ .
The area bounded by the curve y = x4 − 2x3 + x2 + 3 with x-axis and ordinates corresponding to the minima of y is _________ .
The area bounded by the curve y = 4x − x2 and the x-axis is __________ .
The area bounded by the curve y = x |x| and the ordinates x = −1 and x = 1 is given by
Find the coordinates of a point of the parabola y = x2 + 7x + 2 which is closest to the straight line y = 3x − 3.
Find the area of the region bounded by the parabola y2 = 2x and the straight line x – y = 4.
Find the area bounded by the curve y = `sqrt(x)`, x = 2y + 3 in the first quadrant and x-axis.
Find the area of the region bounded by the curve y2 = 2x and x2 + y2 = 4x.
Area of the region bounded by the curve y = cosx between x = 0 and x = π is ______.
The area of the region bounded by the line y = 4 and the curve y = x2 is ______.
The curve x = t2 + t + 1,y = t2 – t + 1 represents
If a and c are positive real numbers and the ellipse `x^2/(4c^2) + y^2/c^2` = 1 has four distinct points in common with the circle `x^2 + y^2 = 9a^2`, then
Find the area of the region bounded by `x^2 = 4y, y = 2, y = 4`, and the `y`-axis in the first quadrant.
Smaller area bounded by the circle `x^2 + y^2 = 4` and the line `x + y = 2` is.
Find the area of the region enclosed by the curves y2 = x, x = `1/4`, y = 0 and x = 1, using integration.
The area enclosed by y2 = 8x and y = `sqrt(2x)` that lies outside the triangle formed by y = `sqrt(2x)`, x = 1, y = `2sqrt(2)`, is equal to ______.
Let a and b respectively be the points of local maximum and local minimum of the function f(x) = 2x3 – 3x2 – 12x. If A is the total area of the region bounded by y = f(x), the x-axis and the lines x = a and x = b, then 4A is equal to ______.
Find the area of the following region using integration ((x, y) : y2 ≤ 2x and y ≥ x – 4).
