Question

Find the area bounded by the curve y = sin x between x = 0 and x = 2π.

Answer 1

Some points on the graph of y = sin x are as follows. The graph is obtained by joining these points with a curve.

x	0	`pi/6`	`pi/4`	`pi/3`	`pi/2`	`(5pi)/6`	`(3pi)/4`	`(2pi)/3`	`pi`
y	0	0.5	0.7	0.8	1	0.5	0.7	0.8	0

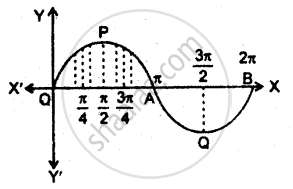
Area of ​​the required region

= Area of ​​the region bounded by the curve OPAQB and the x-axis

= Area of ​​sector OPA + Area of ​​sector AOB

= 2 Area of ​​sector OPA

`= 2 int_0^pi sin x  dx`

`= 2 [- cos x]_0^pi`

= 2[1 + 1]

= 2 × 2

= 4 square unit