Advertisements
Advertisements
Question
The area of the region bounded by the curve y = `sqrt(16 - x^2)` and x-axis is ______.
Options
8 sq.units
20π sq.units
16π sq.units
256π sq.units
Solution
The area of the region bounded by the curve y = `sqrt(16 - x^2)` and x-axis is 8 sq.units.
Explanation:
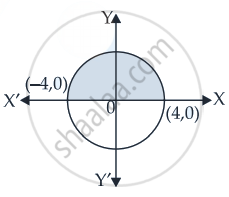
Here, equation of curve is y = `sqrt(16 - x^2)`
Required area = `2[int_0^4 sqrt(16 - x^2) "d"x]`
= `2[x/2 sqrt(16 - x^2) + 16/2 sin^-1 x/4]_0^4`
= `2[(0 + 8 sin^-1 4/4) - (0 + 0)]`
= `2[8sin^-1 (1)]`
= `16 * pi/2`
= 8 sq.units.
APPEARS IN
RELATED QUESTIONS
Draw a rough sketch of the curve and find the area of the region bounded by curve y2 = 8x and the line x =2.
Find the area of the minor segment of the circle \[x^2 + y^2 = a^2\] cut off by the line \[x = \frac{a}{2}\]
Find the area enclosed by the curve x = 3cost, y = 2sin t.
Find the area of the region bounded by x2 = 4ay and its latusrectum.
Find the area of the region bounded by x2 + 16y = 0 and its latusrectum.
Find the area bounded by the curve y = 4 − x2 and the lines y = 0, y = 3.
Using integration, find the area of the triangular region, the equations of whose sides are y = 2x + 1, y = 3x+ 1 and x = 4.
Find the area enclosed by the parabolas y = 5x2 and y = 2x2 + 9.
Sketch the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 1. Also, find the area of this region.
Find the area bounded by the lines y = 4x + 5, y = 5 − x and 4y = x + 5.
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using horizontal strips.
The area bounded by y = 2 − x2 and x + y = 0 is _________ .
The area bounded by the parabola x = 4 − y2 and y-axis, in square units, is ____________ .
The area of the region bounded by the parabola y = x2 + 1 and the straight line x + y = 3 is given by
The area bounded by the parabola y2 = 8x, the x-axis and the latusrectum is ___________ .
Area of the region bounded by the curve y2 = 4x, y-axis and the line y = 3, is
Using integration, find the area of the region bounded by the line x – y + 2 = 0, the curve x = \[\sqrt{y}\] and y-axis.
Draw a rough sketch of the curve y2 = 4x and find the area of region enclosed by the curve and the line y = x.
Find the area of the region bound by the curves y = 6x – x2 and y = x2 – 2x
Find the area of the region bounded by the curve ay2 = x3, the y-axis and the lines y = a and y = 2a.
The area enclosed by the ellipse `x^2/"a"^2 + y^2/"b"^2` = 1 is equal to ______.
Compute the area bounded by the lines x + 2y = 2, y – x = 1 and 2x + y = 7.
Area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2 = 32 is ______.
The region bounded by the curves `x = 1/2, x = 2, y = log x` and `y = 2^x`, then the area of this region, is
Find the area of the region bounded by the ellipse `x^2/4 + y^2/9` = 1.
Find the area bounded by the curve y = |x – 1| and y = 1, using integration.
Let f(x) be a non-negative continuous function such that the area bounded by the curve y = f(x), x-axis and the ordinates x = `π/4` and x = `β > π/4` is `(βsinβ + π/4 cos β + sqrt(2)β)`. Then `f(π/2)` is ______.
Let g(x) = cosx2, f(x) = `sqrt(x)`, and α, β (α < β) be the roots of the quadratic equation 18x2 – 9πx + π2 = 0. Then the area (in sq. units) bounded by the curve y = (gof)(x) and the lines x = α, x = β and y = 0, is ______.
Using integration, find the area of the region bounded by line y = `sqrt(3)x`, the curve y = `sqrt(4 - x^2)` and Y-axis in first quadrant.
Find the area of the minor segment of the circle x2 + y2 = 4 cut off by the line x = 1, using integration.
