Question

Draw a rough sketch of the region {(x, y) : y² ≤ 5x, 5x² + 5y² ≤ 36} and find the area enclosed by the region using method of integration.

Answer 1

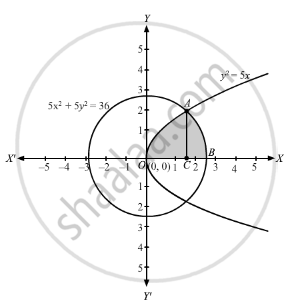
The given region is intersection of\[y^2 \leq 5x\text{ and }5 x^2 + 5 y^2 \leq 36\]

Clearly, \[y^2 \leq 5x\] is a parabola with vertex at origin and the axis is along the x-axis opening in the positive direction. Also \[5 x^2 + 5 y^2 \leq 36\] is a circle with centre at the origin and has a radius \[\sqrt{\frac{36}{5}}\text{ or }\frac{6}{\sqrt{5}}\]

Corresponding equations of given inequations are

\[y^2 = 5x . . . . . \left( 1 \right) \]

\[5 x^2 + 5 y^2 = 36 . . . . . \left( 2 \right)\]

Substituting the value of y² from (1) into (2), we get

\[5 x^2 + 25x = 36\]

\[\Rightarrow 5 x^2 + 25x - 36 = 0\]

\[\Rightarrow x = \frac{- 25 \pm \sqrt{625 + 720}}{10}\]

\[\Rightarrow x = \frac{- 25 \pm \sqrt{1345}}{10}\]

From the figure we see that x-coordinate of intersecting point can not be negative.

\[\therefore x = \frac{- 25 + \sqrt{1345}}{10}\]

Now assume that x-coordinate of intersecting point, \[a = \frac{- 25 + \sqrt{1345}}{10}\]

The  Required area, A  = 2(Area of OACO + Area of CABC)

Approximating the area of OACO  the length = | y₁ |and a width = dx

\[\text{ Area of OACO }= \int_0^a \left| y_1 \right| d x\]

\[= \int_0^a y_1 d x\]

\[= \int_0^a \sqrt{5x} d x ............\left( \because {y_1}^2 = 5x \Rightarrow y_1 = \sqrt{5x} \right)\]

\[\sqrt{5} \left[ \frac{2 x^\frac{3}{2}}{3} \right]^a_0\]

Therefore, Area of OACO \[= \frac{2\sqrt{5} a^\frac{3}{2}}{3}\]

Similarly approximating the area of CABC the length \[=\left| y_2 \right|\]  and the width = dx

\[\text{Area of CABC }= \int_a^\frac{6}{\sqrt{5}} \left| y_2 \right| d x\]

\[= \int_a^\frac{6}{\sqrt{5}} y_2 d x\]

\[= \int_a^\frac{6}{\sqrt{5}} \sqrt{\frac{36}{5} - x^2} d x\]

\[= \left[ \frac{x}{2}\sqrt{\frac{36}{5} - x^2} + \frac{18}{5} \sin^{- 1} \left( \frac{x\sqrt{5}}{6} \right) \right]_a^\frac{6}{\sqrt{5}}\]

\[= \frac{6}{2\sqrt{5}}\sqrt{\frac{36}{5} - \frac{36}{5}} + \frac{18}{5} \sin^{- 1} \left( 1 \right) - \frac{a}{2}\sqrt{\frac{36}{5} - a^2} - \frac{18}{5} \sin^{- 1} \left( \frac{a\sqrt{5}}{6} \right)\]

\[= 0 + \frac{18}{5} \sin^{- 1} \left( 1 \right) - \frac{a}{2}\sqrt{\frac{36}{5} - a^2} - \frac{18}{5} \sin^{- 1} \left( \frac{a\sqrt{5}}{6} \right)\]

\[= \frac{18}{5} \times \frac{\pi}{2} - \frac{a}{2}\sqrt{\frac{36}{5} - a^2} - \frac{18}{5} \sin^{- 1} \left( \frac{a\sqrt{5}}{6} \right)\]
Area of CABC\[= \frac{9\pi}{5} - \frac{a}{2}\sqrt{\frac{36}{5} - a^2} - \frac{18}{5} \sin^{- 1} \left( \frac{a\sqrt{5}}{6} \right)\]
Thus the  Required area, A  = 2(Area of OACO + Area of CABC)

\[A = 2 \left[ \frac{2\sqrt{5} a^\frac{3}{2}}{3} + \frac{9\pi}{5} - \frac{a}{2}\sqrt{\frac{36}{5} - a^2} - \frac{18}{5} \sin^{- 1} \left( \frac{a\sqrt{5}}{6} \right) \right]\] 

\[= \frac{4\sqrt{5} a^\frac{3}{2}}{3} + \frac{18\pi}{5} - a\sqrt{\frac{36}{5} - a^2} - \frac{36}{5} \sin^{- 1} \left( \frac{a\sqrt{5}}{6} \right)\]

\[Where, a = \frac{- 25 + \sqrt{1345}}{10}\]