Advertisements
Advertisements
प्रश्न
If ∠A and ∠P are acute angles such that tan A = tan P, then show that ∠A = ∠P.
उत्तर
A and P are acute angle tan A = tan P
Let us consider right angled triangle ACP,
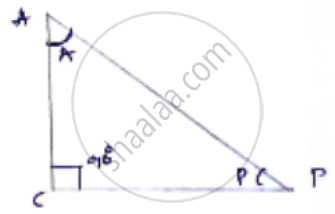
We know `tan theta = "opposite side"/"adjacent side"`
`tan A = (PC)/(AC)`
`tan P = (AC)/(PC)`
tanA =tan P ....(Given)
`(PC)/(AC) = (AC)/(PC)`
`(PC)^2 = (AC)^2`
PC = AC [∵ Angle opposite to equal sides are equal]
∠P = ∠A
APPEARS IN
संबंधित प्रश्न
In a ΔABC , ∠B = 90° , AB = 12 cm and BC = 5 cm Find
(i) cos A (ii) cosec A (iii) cos C (iv) cosec C
If ∠A and ∠B are acute angles such that sin A = Sin B prove that ∠A = ∠B.
`(cos 28°)/(sin 62°)` = ?
In a right-angled triangle, it is given that A is an acute angle and tan A = `(5) /(12)`.
find the value of :
(i) cos A
(ii) sin A
(iii) ` (cosA+sinA)/(cosA– sin A)`
Given : 17 cos θ = 15;
Find the value of: tan θ + 2 secθ .
If sin A = `(sqrt3)/(2)` and cos B = `(sqrt3)/(2)` , find the value of : `(tan"A" – tan"B")/(1+tan"A" tan"B")`
If sec A = `sqrt2` , find : `(3cot^2 "A"+ 2 sin^2 "A")/ (tan^2 "A" – cos ^2 "A")`.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
sinA = `(12)/(13)`
If tan = 0.75, find the other trigonometric ratios for A.
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cosec C
