Advertisements
Advertisements
प्रश्न
In a ΔABC , ∠B = 90° , AB= 24 cm and BC = 7 cm find (i) sin A (ii) cos A (iii) sin C (iv) cos C
उत्तर
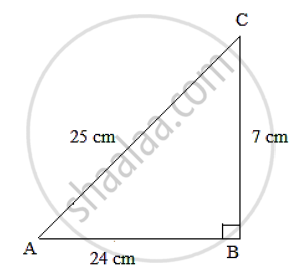
Using Pythagoras theorem, we get:
`AC^2 = AB^2 + BC^2`
`⟹ AC^2 = (24)^2 + (7)^2`
`⟹ AC^2 = 576 + 49 = 625`
⟹ 𝐴𝐶 = 25 𝑐𝑚
Now, for T-Ratios of ∠𝐴, 𝑏𝑎𝑠𝑒 = 𝐴𝐵 𝑎𝑛𝑑 𝑝𝑒𝑟𝑝𝑒𝑛𝑑𝑖𝑐𝑢𝑙𝑎𝑟 = 𝐵𝐶
(i) sin A = `(BC)/(AC) = 7/25`
(ii) cos A = `(AB)/(AC)=24/25`
Similarly, for T-Ratios of ∠𝐶, 𝑏𝑎𝑠𝑒 = 𝐵𝐶 𝑎𝑛𝑑 𝑝𝑒𝑟𝑝𝑒𝑛𝑑𝑖𝑐𝑢𝑙𝑎𝑟 = 𝐴𝐵
(iii) sin C = `(AB)/(AC)=24/25`
(iv) cos C= `(BC)/(AC)=7/25`
APPEARS IN
संबंधित प्रश्न
If ∠A and ∠P are acute angles such that tan A = tan P, then show that ∠A = ∠P.
f θ = 30°, verify that cos 3θ = 4 cos3 θ − 3 cos θ
If cos 2θ = sin 4θ where 2θ, 4θ are acute angles, find the value of θ.
If sin ∝ = `1/2` prove that (3cos∝ - `4cos^2` ∝)=0
If ∠A and ∠B are acute angles such that tan A= Tan B then prove that ∠A = ∠B
Evaluate:
cos600 cos300− sin600 sin300
If A = 300 , verify that:
(i) sin 2A = `(2 tan A)/(1+tan^2A)`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
tanB = `(8)/(15)`
If cosec θ = `(29)/(20)`, find the value of: `("sec" θ)/("tan" θ - "cosec" θ)`
From the given figure, prove that θ + ∅ = 90°. Also prove that there are two other right angled triangles. Find sin α, cos β and tan ∅
