Advertisements
Advertisements
प्रश्न
Prove that
cosec (65 °+ θ) sec (25° − θ) − tan (55° − θ) + cot (35° + θ) = 0
उत्तर
\[\begin{array}{l}\text{ L.H.S}=cosec( {65}^0 + \theta) - \sec( {25}^0 - \theta) - \tan( {55}^0 - \theta) + \cot( {35}^0 + \theta) \\ \end{array}\]
\[\begin{array}{l}= \ cosec{ {90}^0 - ( {25}^0 - \theta)} - \sec( {25}^0 - \theta) - \tan( {55}^0 - \theta) + \cot{ {90}^0 -( {55}^0 - \theta)} \\ \end{array}\]
\[\begin{array}{l}= \sec( {25}^0 - \theta) - \sec( {25}^0 - \theta) - \tan( {55}^0 - \theta) + \tan( {55}^0 -\theta) \\ \end{array}\]
= 0
= RHS
APPEARS IN
संबंधित प्रश्न
if `cos theta = 5/13` find the value of `(sin^2 theta - cos^2 theta)/(2 sin theta cos theta) = 3/5`
If cosec θ= 2 show that `(cot θ +sin θ /(1+cos θ )) =2`
If x = cosec A +cos A and y = cosec A – cos A then prove that `(2/(x+y))^2 + ((x-y)/2)^2` = 1
Evaluate:
`2cos^2 60^0+3 sin^2 45^0 - 3 sin^2 30^0 + 2 cos^2 90 ^0`
In the adjoining figure, ΔABC is a right-angled triangle in which ∠B = 900, ∠300 and AC = 20cm. Find (i) BC, (ii) AB.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
tan C = `(5)/(12)`
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of cos y
If sin A = `(7)/(25)`, find the value of : `"cos A" + (1)/"cot A"`
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: sin A cos A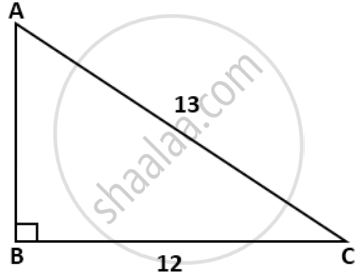
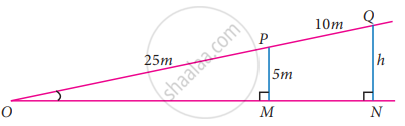
A boy standing at a point O finds his kite flying at a point P with distance OP = 25 m. It is at a height of 5 m from the ground. When the thread is extended by 10 m from P, it reaches a point Q. What will be the height QN of the kite from the ground? (use trigonometric ratios)