Advertisements
Advertisements
प्रश्न
If cosec θ= 2 show that `(cot θ +sin θ /(1+cos θ )) =2`
उत्तर
Let us consider a right ΔABC, right angled at B and ∠𝐶 = 𝜃.
Now, it is given that cosec θ = 2.
Also , sin θ ` = 1/(cosecθ) = 1/2 = (AB)/(AC)`
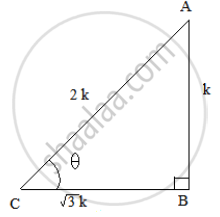
So, if AB =k, then AC =2k, where k is a positive number.
Using Pythagoras theorem, we have:
`⟹ AC^2 = AB^2 + BC^2`
`⟹ BC^2 = AC^2 − AB^2`
`⟹ BC^2 (2K)^2 − (K)^2`
`⟹ BC^2 = 3K^2`
`⟹ BC = sqrt(3k)`
Finding out the other T-ratios using their definitions, we get:
`cos θ = (BC)/(AC) = (sqrt(3k))/(2k) = (sqrt(3))/2`
`tan θ = (AB)/(BC) = K/(sqrt(3k)) = 1/(sqrt(3))`
`Cot θ = 1/ (tan θ) = sqrt(3)`
Substituting these values in the given expression, we get:
cot θ +`(sin θ)/(1+cos θ)`
`= sqrt(3)+((1/2))/(1+sqrt(3)/2`
=`sqrt(3) + (1/2)/((2+sqrt(3))/2)`
=`sqrt(3) + 1/(2+sqrt(3)`
=`(sqrt(3)(2+sqrt(3)+1))/(2+sqrt(3))`
=`(2sqrt(3)+3+1)/(2+sqrt(3)`
=` (2(2+sqrt(3)))/(2+sqrt(3))=2`
i.e., LHS = RHS
Hence proved.
APPEARS IN
संबंधित प्रश्न
If 8 tan A = 15, find sin A – cos A.
If A = B = 60°. Verify `tan (A - B) = (tan A - tan B)/(1 + tan tan B)`
In right angled triangle ΔABC at B, ∠A = ∠C. Find the values of sin A sin B + cos A cos B
If sec θ = `5/4 ` show that `((sin θ - 2 cos θ))/(( tan θ - cot θ)) = 12/7`
If 3tan θ 4 , show that `((4cos theta - sin theta ))/((4 cos theta + sin theta))=4/5`
In the figure of ΔPQR , ∠P = θ° and ∠R =∅° find
(i) `sqrt(X +1) cot ∅`
(ii)`sqrt( x^3 + x ^2) tantheta`
(iii) cos θ

From the following figure, find the values of
(i) sin B
(ii) tan C
(iii) sec2 B - tan2B
(iv) sin2C + cos2C
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of sin x
If cosec θ = `(29)/(20)`, find the value of: `("sec" θ)/("tan" θ - "cosec" θ)`
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: sin A cos A