Advertisements
Advertisements
प्रश्न
The function f is defined by \[f\left( x \right) = \begin{cases}x^2 , & 0 \leq x \leq 3 \\ 3x, & 3 \leq x \leq 10\end{cases}\]
The relation g is defined by \[g\left( x \right) = \begin{cases}x^2 , & 0 \leq x \leq 2 \\ 3x, & 2 \leq x \leq 10\end{cases}\]
Show that f is a function and g is not a function.
उत्तर
The function f is defined by
\[f\left( x \right) = \begin{cases}x^2 & 0 \leqslant x \leqslant 3 \\ 3x & 3 \leqslant x \leqslant 10\end{cases}\]
It is observed that for 0 ≤ x < 3, f (x) = x2.
3 < x ≤ 10, f (x) = 3x
Also, at x = 3, f(x) = 32 = 9. And
f (x) = 3 × 3 = 9.
That is, at x = 3, f (x) = 9.
Therefore, for 0 ≤ x ≤ 10, the images of f (x) are unique.
Thus, the given relation is a function.
Again,
the relation g is defined as
g(x) = 3 × 2 = 6.
Hence, 2 in the domain of the relation g corresponds to two different images, i.e. 4 and 6.
Hence, this relation is not a function.
APPEARS IN
संबंधित प्रश्न
Let A = {9, 10, 11, 12, 13} and let f: A → N be defined by f(n) = the highest prime factor of n. Find the range of f.
Define a function as a correspondence between two sets.
Let A = {−2, −1, 0, 1, 2} and f : A → Z be a function defined by f(x) = x2 − 2x − 3. Find:
(b) pre-images of 6, −3 and 5.
A function f : R → R is defined by f(x) = x2. Determine (a) range of f, (b) {x : f(x) = 4}, (c) [y: f(y) = −1].
If \[y = f\left( x \right) = \frac{ax - b}{bx - a}\] , show that x = f(y).
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iii) f g
If f(x) = cos (log x), then the value of f(x2) f(y2) −
If x ≠ 1 and \[f\left( x \right) = \frac{x + 1}{x - 1}\] is a real function, then f(f(f(2))) is
The domain of definition of the function \[f\left( x \right) = \sqrt{\frac{x - 2}{x + 2}} + \sqrt{\frac{1 - x}{1 + x}}\] is
The domain of the function \[f\left( x \right) = \sqrt{5 \left| x \right| - x^2 - 6}\] is
The range of the function \[f\left( x \right) = \frac{x + 2}{\left| x + 2 \right|}\],x ≠ −2 is
The range of \[f\left( x \right) = \frac{1}{1 - 2\cos x}\] is
If f(m) = m2 − 3m + 1, find f(0)
Check if the following relation is a function.
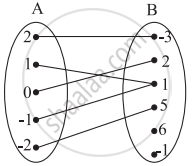
Check if the relation given by the equation represents y as function of x:
x + y2 = 9
Check if the relation given by the equation represents y as function of x:
3x − 6 = 21
If f(m) = m2 − 3m + 1, find f(x + 1)
Find x, if g(x) = 0 where g(x) = `(18 -2x^2)/7`
Solve for x.
log2 x + log4 x + log16 x = `21/4`
The equation logx2 16 + log2x 64 = 3 has,
Select the correct answer from given alternatives.
Let the function f be defined by f(x) = `(2x + 1)/(1 - 3x)` then f–1 (x) is ______.
Select the correct answer from given alternative.
The domain and range of f(x) = 2 − |x − 5| is
Answer the following:
Find whether the following function is one-one
f : R → R defined by f(x) = x2 + 5
Answer the following:
Let f : R → R be given by f(x) = x3 + 1 for all x ∈ R. Draw its graph
Answer the following:
If `log (("a" + "b")/2) = 1/2(log"a" + log"b")`, then show that a = b
Answer the following:
If b2 = ac. prove that, log a + log c = 2 log b
Answer the following:
If a2 = b3 = c4 = d5, show that loga bcd = `47/30`
Answer the following:
Find the domain of the following function.
f(x) = 5–xPx–1
Given the function f: x → x2 – 5x + 6, evaluate f(x – 1)
The data in the adjacent table depicts the length of a person's forehand and their corresponding height. Based on this data, a student finds a relationship between the height (y) and the forehand length (x) as y = ax + b, where a, b are constant.
| Length ‘x’ of forehand (in cm) |
Height 'y' (in inches) |
| 35 | 56 |
| 45 | 65 |
| 50 | 69.5 |
| 55 | 74 |
Find a and b
Let A = {1, 2, 3, 4} and B = N. Let f : A → B be defined by f(x) = x3 then, find the range of f
Mapping f: R → R which is defined as f(x) = sin x, x ∈ R will be ______
Find the range of the following functions given by f(x) = |x − 3|
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find (f + g)(x)
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find (f – g)(x)
The domain of the function f given by f(x) = `(x^2 + 2x + 1)/(x^2 - x - 6)` is ______.
The domain and range of the function f given by f(x) = 2 – |x – 5| is ______.
