Advertisements
Advertisements
Question
Find the area of the unshaded region shown in the given figure.
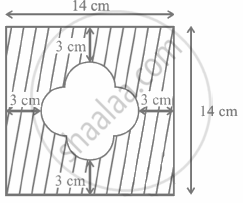
Solution
The side of a square = Diameter of the semi-circle = a
Area of the unshaded region
= Area of a square of side ‘a’ + 4(Area of a semi-circle of diameter ‘a’)
The horizontal/vertical extent of the white region = 14 – 3 – 3 = 8 cm
Radius of the semi-circle + Side of a square + Radius of the semi-circle = 8 cm
2 (radius of the semi-circle) + Side of a square = 8 cm
2a = 8 cm
`\implies` a = 4 cm
Area of the unshaded region
= Area of a square of side 4 cm + 4 (Area of a semi-circle of diameter 4 cm)
= `(4)^2 + 4 xx 1/2 π(2)^2`
= (16 + 8π) cm2
APPEARS IN
RELATED QUESTIONS
A chord 10 cm long is drawn in a circle whose radius is 5√2 cm. Find the area of both
segments
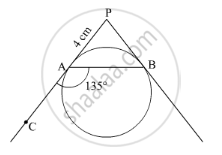
In the given figure, PA and PB are tangents to a circle from an external point P such that PA = 4 cm and ∠BAC = 135°. Find the length of chord AB ?
The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
In the following figure, if m(arc DXE) = 120° and m(arc AYC) = 60°. Find ∠DBE.

The diameter of a sphere is 6 cm, Find the total surface area of the sphere. (π = 3.14)
The area of the shaded portion in the following figure is equal to the area of.
Find the area of the minor segment of a circle of radius 14 cm, when the angle of the corresponding sector is 60°.
Four circular cardboard pieces of radii 7 cm are placed on a paper in such a way that each piece touches other two pieces. Find the area of the portion enclosed between these pieces.
The central angles of two sectors of circles of radii 7 cm and 21 cm are respectively 120° and 40°. Find the areas of the two sectors as well as the lengths of the corresponding arcs. What do you observe?
The area of the sector of a circle of radius 12 cm is 60π cm2. The central angle of this sector is ______.
