Advertisements
Advertisements
Question
Given: tan A = `4/3 , "find" : ("cosec""A")/(cot "A"– sec "A")`
Solution
Consider the diagram below :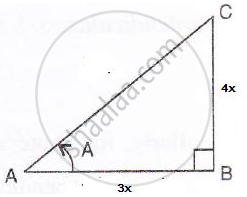
tan A = `(4)/(3)`
i.e. `"perpendicular"/"base" = (4)/(3) ⇒ "BC"/"AB" = (4)/(3)`
Therefore if length of AB = 3x, length of BC = 4x
Since
AB2 + BC2 = AC2 ... [ Using Pythagoras Theorem ]
( 3x )2 + (4x)2 = AC2
AC2 = 9x2 + 16x2 = 25x2
AC = 5x ...( hypotenuse )
Now
sec A = `" hypotenuse "/"base" = "AC"/"AB" =(5x)/ (3x) = (5)/(3)`
cot A = `" base "/"perpendicular" = "AB"/"BC" = (3x)/ (4x) = (3)/(4)`
cosec A = `" hypotenuse "/"perpendicular" = "AC"/"BC" = (5x)/(4x) = (5)/(4)`
Therefore
`("cosec""A")/(cot"A" – sec "A")`
= `(5/ 4) /(3/4 – 5/3)`
= ` (5 /4)/(– 11/12)`
= `– (60)/(44) `
= `– (15)/(11)`
APPEARS IN
RELATED QUESTIONS
If 8 tan A = 15, find sin A – cos A.
If Sin (A + B) = 1 and cos (A – B) = 1, 0° < A + B ≤ 90° A ≥ B. Find A & B
If sin A = `9/41` find all the values of cos A and tan A
Show that:
(i)` (1-sin 60^0)/(cos 60^0)=(tan60^0-1)/(tan60^0+1)`
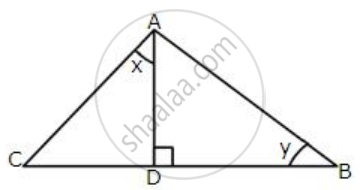
In the following figure:
AD ⊥ BC, AC = 26 CD = 10, BC = 42, ∠DAC = x and ∠B = y.
Find the value of :
(i) cot x
(ii) `1/sin^2 y – 1/tan^2 y`
(iii) `6/cos x – 5/cos y + 8 tan y`.
If cosec A + sin A = 5`(1)/(5)`, find the value of cosec2A + sin2A.
If sin θ = `(8)/(17)`, find the other five trigonometric ratios.
If tan = 0.75, find the other trigonometric ratios for A.
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: cot2P - cosec2P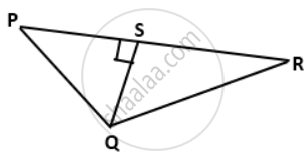
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cos C
