Advertisements
Advertisements
Question
If \[f\left( x \right) = \frac{x + 1}{x - 1}\] , show that f[f[(x)]] = x.
Solution
Given:
\[f\left( x \right) = \frac{x + 1}{x - 1}\]
Therefore,
\[f\left[ f\left\{ \left( x \right) \right\} \right] = f\left( \frac{x + 1}{x - 1} \right)\]
f [ f {(x)}] = x
Hence proved.
APPEARS IN
RELATED QUESTIONS
What is the fundamental difference between a relation and a function? Is every relation a function?
f, g, h are three function defined from R to R as follow:
(i) f(x) = x2
Find the range of function.
Let X = {1, 2, 3, 4} and Y = {1, 5, 9, 11, 15, 16}
Determine which of the set are functions from X to Y.
(a) f1 = {(1, 1), (2, 11), (3, 1), (4, 15)}
If f : R → R be defined by f(x) = x2 + 1, then find f−1 [17] and f−1 [−3].
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iv) \[\frac{f}{g}\]
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(i) f + g
Let f and g be two real functions given by
f = {(0, 1), (2, 0), (3, −4), (4, 2), (5, 1)} and g = {(1, 0), (2, 2), (3, −1), (4, 4), (5, 3)}
Find the domain of fg.
Let f : R → R be defined by f(x) = 2x + |x|. Then f(2x) + f(−x) − f(x) =
Let A = {x ∈ R : x ≠ 0, −4 ≤ x ≤ 4} and f : A ∈ R be defined by \[f\left( x \right) = \frac{\left| x \right|}{x}\] for x ∈ A. Then th (is
If \[3f\left( x \right) + 5f\left( \frac{1}{x} \right) = \frac{1}{x} - 3\] for all non-zero x, then f(x) =
If f : R → R be given by for all \[f\left( x \right) = \frac{4^x}{4^x + 2}\] x ∈ R, then
The domain of definition of the function \[f\left( x \right) = \sqrt{x - 1} + \sqrt{3 - x}\] is
The domain of definition of the function f(x) = log |x| is
The range of the function \[f\left( x \right) = \frac{x + 2}{\left| x + 2 \right|}\],x ≠ −2 is
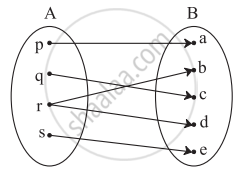
Check if the following relation is a function.
Find x, if g(x) = 0 where g(x) = `(18 -2x^2)/7`
Find the domain and range of the following function.
f(x) = `root(3)(x + 1)`
If `log(( x - y)/4) = logsqrt(x) + log sqrt(y)`, show that (x + y)2 = 20xy
If f(x) = 3x + 5, g(x) = 6x − 1, then find `("f"/"g") (x)` and its domain
Select the correct answer from given alternatives.
If log10(log10(log10x)) = 0 then x =
Select the correct answer from given alternatives.
Find x, if 2log2 x = 4
Answer the following:
Find whether the following function is one-one
f : R → R defined by f(x) = x2 + 5
Answer the following:
Find whether the following function is one-one
f : R − {3} → R defined by f(x) = `(5x + 7)/(x - 3)` for x ∈ R − {3}
Answer the following:
Let f: R → R be a function defined by f(x) = 5x3 – 8 for all x ∈ R, show that f is one-one and onto. Hence find f –1
Answer the following:
If f(x) = 3x + a and f(1) = 7 find a and f(4)
Answer the following:
If `log"a"/(x + y - 2z) = log"b"/(y + z - 2x) = log"c"/(z + x - 2y)`, show that abc = 1
Answer the following:
Show that, logy x3 . logz y4 . logx z5 = 60
Answer the following:
If a2 = b3 = c4 = d5, show that loga bcd = `47/30`
Answer the following:
Find the domain of the following function.
f(x) = x!
Let X = {3, 4, 6, 8}. Determine whether the relation R = {(x, f(x)) | x ∈ X, f(x) = x2 + 1} is a function from X to N?
A graph representing the function f(x) is given in it is clear that f(9) = 2
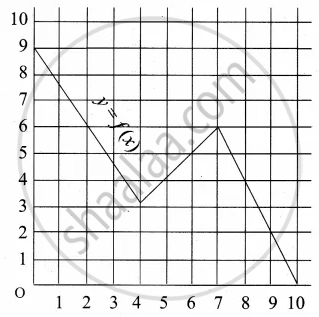
For what value of x is f(x) = 1?
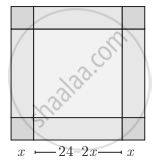
An open box is to be made from a square piece of material, 24 cm on a side, by cutting equal square from the corner and turning up the side as shown. Express the volume V of the box as a function of x
The domain of the function f(x) = `sqrtx` is ______.
The range of the function f(x) = `(x - 3)/(5 - x)`, x ≠ 5 is ______.
Redefine the function f(x) = x − 2 + 2 + x , – 3 ≤ x ≤ 3
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find (f + g)(x)
If f(x) = y = `(ax - b)/(cx - a)`, then prove that f(y) = x.
The domain and range of the real function f defined by f(x) = `(4 - x)/(x - 4)` is given by ______.
The domain for which the functions defined by f(x) = 3x2 – 1 and g(x) = 3 + x are equal is ______.
Let f(θ) = sin θ (sin θ + sin 3θ) then ______.
