Advertisements
Advertisements
Question
If the side BC of ΔABC is produced on both sides, then write the difference between the sum of the exterior angles so formed and ∠A.
Solution
In the given problem, we need to find the difference between the sum of the exterior angles and∠A.
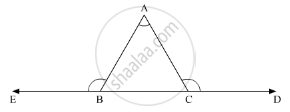
Now, according to the exterior angle theorem
ext. ∠C = ∠A + ∠B .........(1)
Also,
ext. ∠B = ∠A + ∠C .........(2)
Further, adding (1) and (2)
ext. ∠C + ext. ∠B = ∠A + ∠B + ∠A + ∠B
=2∠A+ ∠B + ∠C .........(3)
Also, according to the angle sum property of the triangle, we get,
∠A + ∠B + ∠C = 180° .........(4)
Now, we need to find the difference between the sum of the exterior angles and ∠A
Thus,
( ext. ∠C + ext. ∠B) - ∠A = (2∠A + ∠B + ∠C ) - ∠A
= ∠A = ∠B + ∠C
= 180°(Using 4)
Therefore, (ext. ∠C ext.∠B) -∠A = 180°
APPEARS IN
RELATED QUESTIONS
Can a triangle have two right angles? Justify your answer in case.
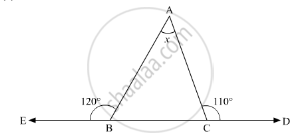
Compute the value of x in the following figure:
Is the following statement true and false :
A triangle can have at most one obtuse angles.
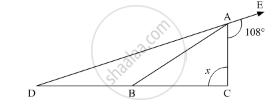
In the given figure, AB divides ∠DAC in the ratio 1 : 3 and AB = DB. Determine the value of x.
In Δ ABC, if u∠B = 60°, ∠C = 80° and the bisectors of angles ∠ABC and ∠ACB meet at a point O, then find the measure of ∠BOC.
Side BC of a triangle ABC has been produced to a point D such that ∠ACD = 120°. If ∠B = \[\frac{1}{2}\]∠A is equal to
State, if the triangle is possible with the following angles :
40°, 130°, and 20°
Find the unknown marked angles in the given figure:

In ∆ABC, ∠A = ∠B = 62° ; find ∠C.
Find all the three angles of the ΔABC
