Advertisements
Advertisements
Question
If sin x + cos y = 1 and x = 30°, find the value of y
Solution
Given that x = 30°
sin x + cos y = 1
sin 30° + cos y = 1
cos y = 1 – sin 30°
cos y = 1 –`(1)/(2)`
cos y = `(1)/(2)`
cos y = cos 60°
y = 60°
APPEARS IN
RELATED QUESTIONS
Calculate the value of A, if (sin A - 1) (2 cos A - 1) = 0
If θ = 30°, verify that: sin2θ = `(2tanθ)/(1 ++ tan^2θ)`
If `sqrt(3)`sec 2θ = 2 and θ< 90°, find the value of θ
Find:
a. BC
b. AD
c. AC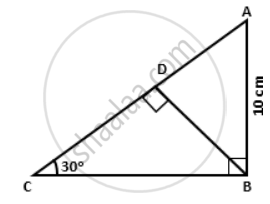
Find the value 'x', if: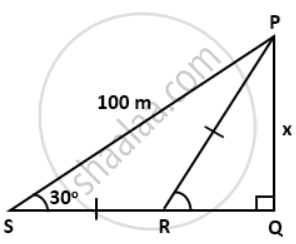
In the given figure, if tan θ = `(5)/(13), tan α = (3)/(5)` and RS = 12m, find the value of 'h'.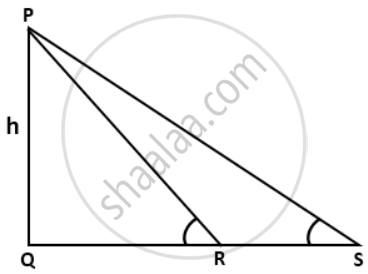
The perimeter of a rhombus is 100 cm and obtuse angle of it is 120°. Find the lengths of its diagonals.
Evaluate the following: sec16° tan28° - cot62° cosec74°
Evaluate the following: sin(35° + θ) - cos(55° - θ) - tan(42° + θ) + cot(48° - θ)
If A, B and C are interior angles of ΔABC, prove that sin`(("A" + "B")/2) = cos "C"/(2)`
