Advertisements
Advertisements
Question
If tan θ =`15/ 8 `, find the values of all T-ratios of θ.
Solution
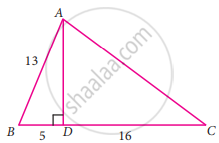
Let us first draw a right ΔABC, right angled at B and ∠𝐶 = 𝜃
Now, we know that tan θ = `"𝑃𝑒𝑟𝑝𝑒𝑛𝑑𝑖𝑐𝑢𝑙𝑎𝑟"/" 𝐵𝑎𝑠𝑒"=(AB)/(BC) = 15/8`
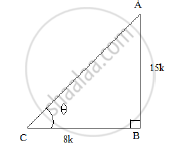
So, if BC = 8k, then AB = 15k where k is positive number.
Now, using Pythagoras theorem, we have:
`AC^2 = AB^2 + BC^2 = (15K)^2 + (8K)^2`
`⟹ AC^2 = 225K^2 + 64^2 = 289^2`
⟹ AC = 17k
Now, finding the other T-ratios using their definitions, we get:
Sin 𝜃 = `(AB)/(AC) = (15 K)/(17K) = 15/17`
Cos θ = `(BC)/(AC) = (8K)/(17K) = 8/17`
∴ cot 𝜃 = `1/(tan θ ) = 8/15 , cosec θ = 1/(sin θ ) = 17/15 and sec θ = 1/(cos θ ) = 17/8`
APPEARS IN
RELATED QUESTIONS
if `sin theta = 3/5 " evaluate " (cos theta - 1/(tan theta))/(2 cot theta)`
If ∠A and ∠P are acute angles such that tan A = tan P, then show that ∠A = ∠P.
If cosec θ = `sqrt(10)` find all the values of all T-ratios of θ
If tan θ = `20/21` show that `((1-sin θ + cos θ))/((1+ sin θ +cos θ)) = 3/7`
From the following figure, find the values of :
(i) sin A
(ii) sec A
(iii) cos2 A + sin2A
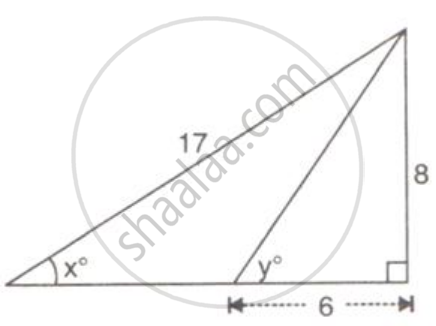
Use the given figure to find :
(i) sin xo
(ii) cos yo
(iii) 3 tan xo - 2 sin yo + 4 cos yo.
If sinA = `(3)/(5)`, find cosA and tanA.
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: cot2P - cosec2P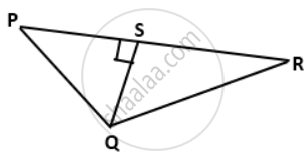
From the given figure, find the values of sin B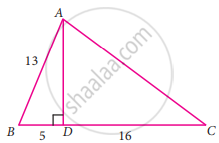
From the given figure, find the values of cot B