Advertisements
Advertisements
Question
In the following figure, ABC is an equilateral triangle in which QP is parallel to AC. Side AC is produced up to point R so that CR = BP.
Prove that QR bisects PC.
Hint: ( Show that ∆ QBP is equilateral
⇒ BP = PQ, but BP = CR
⇒ PQ = CR ⇒ ∆ QPM ≅ ∆ RCM ).
Solution
ΔABC is an equilateral triangle,
So, each of its angles equals 60°.
QP is parallel to AC,
⇒ ∠PQB = ∠RAQ = 60°
ln ΔQBP,
∠PQB = ∠BQP = 60°
So, ∠PBQ + ∠BQP + ∠BPQ = 180° ....(angle sum property)
⇒ 60°+ 60° + ∠BPQ = 180°
⇒ ∠BPQ = 60°
So, ΔBPQ is an equilateral triangle.
⇒ QP = BP
⇒ QP = CR ....(i)
Now, ∠QPM + ∠BPQ = 180° ...(linear pair)
⇒ ∠QPM+ 60°= 180°
⇒ ∠QPM = 120°
Also, ∠RCM+ ∠ACB = 180° ...(linear pair)
⇒ ∠RCM+ 60° = 180°
⇒ ∠RCM = 120°
ln ΔRCM and ΔQMP,
∠RCM = ∠QPM ....(each is 120°)
∠RMC = ∠QMP ...(vertically opposite angles)
QP= CR ....(from(i))
⇒ ΔRCM ≅ ΔQMP ....(AAS congruence criterion)
So, CM = PM
⇒ QR bisects PC.
APPEARS IN
RELATED QUESTIONS
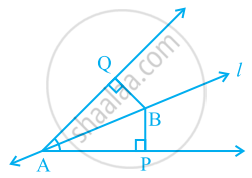
Line l is the bisector of an angle ∠A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠A (see the given figure). Show that:
- ΔAPB ≅ ΔAQB
- BP = BQ or B is equidistant from the arms of ∠A.
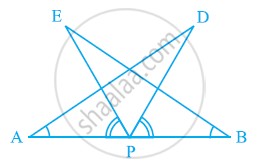
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB (See the given figure). Show that
- ΔDAP ≅ ΔEBP
- AD = BE
Which congruence criterion do you use in the following?
Given: EB = DB
AE = BC
∠A = ∠C = 90°
So, ΔABE ≅ ΔCDB

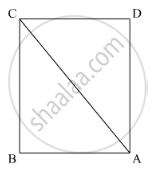
In the given figure, prove that:
CD + DA + AB + BC > 2AC
If the following pair of the triangle is congruent? state the condition of congruency :
In Δ ABC and Δ DEF, AB = DE, BC = EF and ∠ B = ∠ E.
The following figure shows a circle with center O.
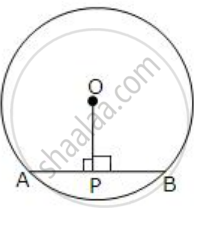
If OP is perpendicular to AB, prove that AP = BP.
In a triangle ABC, D is mid-point of BC; AD is produced up to E so that DE = AD. Prove that:
AB is parallel to EC.
The perpendicular bisectors of the sides of a triangle ABC meet at I.
Prove that: IA = IB = IC.
In the following figure, BL = CM.

Prove that AD is a median of triangle ABC.
In a triangle, ABC, AB = BC, AD is perpendicular to side BC and CE is perpendicular to side AB.
Prove that: AD = CE.
