Advertisements
Advertisements
Question
O is the point of intersection of the diagonals AC and BD of a trapezium ABCD with AB || DC. Through O, a line segment PQ is drawn parallel to AB meeting AD in P and BC in Q. Prove that PO = QO.
Solution
Given ABCD is a trapezium.
Diagonals AC and BD are intersect at O.
PQ || AB || DC
To prove: PO = QO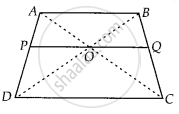
Proof: In ∆ABD and ∆POD,
PO || AB ...[∵ PQ || AB]
∠D = ∠D ...[Common angle]
∠ABD = ∠POD ...[Corresponding angles]
∴ ∆ABD ~ ∆POD ...[By AAA similarity criterion]
Then, `("OP")/("AB") = ("PD")/("AD")` ...(i)
In ∆ABC and ∆OQC,
OQ || AB ...[∵ OQ || AB]
∠C = ∠C ...[Common angle]
∠BAC = ∠QOC ...[Corresponding angles]
∴ ∆ABC ~ ∆OQC ...[By AAA similarity criterion]
Then, `("OQ")/("AB") = ("QC")/("BC")` ...(ii)
Now, In ∆ADC,
OP || DC
∴ `("AP")/("PD") = ("OA")/("OC")` [By basic proportionality theorem] ...(iii)
In ∆ABC,
OQ || AB
∴ `("BQ")/("QC") = ("OA")/("OC")` [By basic proportionality theorem] ...(iv)
From equations (iii) and (iv), we get
`("AP")/("PD") = ("BQ")/("QC")`
Adding 1 on both sides, we get
`("AP")/("PD") + 1 = ("BQ")/("QC") + 1`
⇒ `("AP" + "PD")/("PD") = ("BQ" + "QC")/("QC")`
⇒ `("AD")/("PD") = ("BC")/("QC")`
⇒ `("PD")/("AD") = ("QC")/("BC")`
⇒ `("OP")/("AB") = ("OQ")/("BC")` ...[From equations (i) and (ii)]
⇒ `("OP")/("AB") = ("OQ")/("AB")` ...[From equation (ii)]
⇒ OP = OQ
Hence proved.
APPEARS IN
RELATED QUESTIONS
In the given figure, PS is the bisector of ∠QPR of ΔPQR. Prove that `(QS)/(SR) = (PQ)/(PR)`

In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 8x − 7, DB = 5x − 3, AE = 4x − 3 and EC = (3x − 1), find the value of x.
M and N are points on the sides PQ and PR respectively of a ΔPQR. For the following case, state whether MN || QR
PM = 4cm, QM = 4.5 cm, PN = 4 cm and NR = 4.5 cm
In the given figure, ABCD is a trapezium in which AB║DC and its diagonals intersect at O. If AO = (5x – 7), OC = (2x + 1) , BO = (7x – 5) and OD = (7x + 1), find the value of x.

ΔABC is an isosceles triangle with AB = AC = 13cm. The length of altitude from A on BC is 5cm. Find BC.
State the basic proportionality theorem.
Draw an isosceles triangle with base 5 cm and height 4 cm. Draw a triangle similar to the triangle drawn whose sides are `2/3` times the sides of the triangle.
Prove that, if a line parallel to a side of a triangle intersects the other sides in two district points, then the line divides those sides in proportion.
In fig, seg DE || sec BC, identify the correct statement.
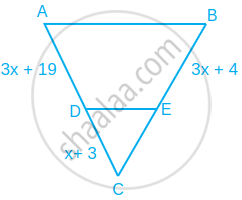
Find the value of x for which DE || AB in figure.