Advertisements
Advertisements
Question
Prove that the quadrilateral formed by angle bisectors of a cyclic quadrilateral ABCD is also cyclic.
Solution
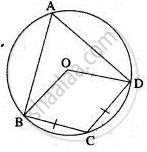
Given: In cyclic ABCD the bisectors formed a quadrilateral ABCD.
To prove: PQRS is a cyclic quadrilateral.
Proof: In cyclic quadrilateral ABCD, AR and BS be the bisectors of ∠A and ∠B.
So, ∠ 1 = ∠ A/2 and ∠ 2 = ∠ B/2
In Δ ASB, ∠ RSP is the exterior angle
So ∠ RSP = ∠1 + ∠2
∠ RSP = `"∠A"/2 + "∠B"/2` ....(i)
Similarly, ∠ PQR = `"∠C"/2 + "∠D"/2` ....(ii)
Adding (i) and (ii),
∠ PQR + ∠ RSP = `1/2`(∠A + ∠B + ∠C + ∠D)
= `1/2` x 360° = 180°
∠ PQR + ∠ RSP = 180°
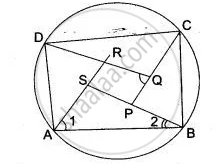
But these are the opposite angles of quadrilateral PQRS
Hence PQRS is a cyclic quadrilateral.
Hence proved.
APPEARS IN
RELATED QUESTIONS
In a cyclic quadrilateral ABCD, the diagonal AC bisects the angle BCD. Prove that the diagonal BD is parallel to the tangent to the circle at point A.
Prove that any four vertices of a regular pentagon are concylic (lie on the same circle).
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°.

Find:
- ∠CAD
- ∠CBD
- ∠ADC
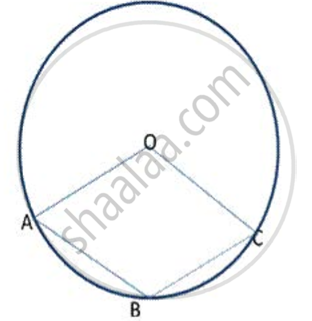
In fig., O is the centre of the circle and ∠ AOC = 1500. Find ∠ ABC.
In triangle ABC, AB = AC. A circle passing through B and c intersects the sides AB and AC at D and E respectively. Prove that DE || BC.
Prove that the angles bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (provided they are not parallel) intersect at right triangle.
In cyclic quadrilateral ABCD, ∠DAC = 27°; ∠DBA = 50° and ∠ADB = 33°. Calculate : ∠CAB.

In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BDC
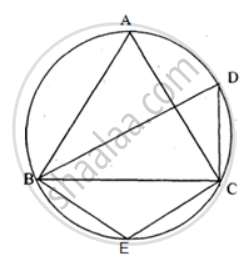
If ABCD is a cyclic quadrilateral in which AD || BC. Prove that ∠B = ∠C.
In the given figure O is the center of the circle, ∠ BAD = 75° and chord BC = chord CD. Find:
(i) ∠BOC (ii) ∠OBD (iii) ∠BCD.