Advertisements
Advertisements
Question
State, if the triangle is possible with the following angles :
125°, 40°, and 15°
Solution
We know that the sum of three angles of a triangle is 180°, therefore
Sum of 125°, 40°, and 15°
= 125° + 40° + 15° = 180°
Since the sum is 180°, therefore it is possible.
APPEARS IN
RELATED QUESTIONS
If each angle of a triangle is less than the sum of the other two, show that the triangle is acute angled.
In Δ ABC, BD⊥ AC and CE ⊥ AB. If BD and CE intersect at O, prove that ∠BOC = 180° − A.
In Δ ABC, if u∠B = 60°, ∠C = 80° and the bisectors of angles ∠ABC and ∠ACB meet at a point O, then find the measure of ∠BOC.
In the given figure, side BC of ΔABC is produced to point D such that bisectors of ∠ABC and ∠ACD meet at a point E. If ∠BAC = 68°, find ∠BEC.

If the sides of a triangle are produced in order, then the sum of the three exterior angles so formed is
In the following, find the marked unknown angle:

Find, giving a reason, the unknown marked angles, in a triangle drawn below:
The length of the sides of the triangle is given. Say what types of triangles they are 3.7 cm, 3.4 cm, 4 cm.
The image of an object placed at a point A before a plane mirror LM is seen at the point B by an observer at D as shown in the following figure. Prove that the image is as far behind the mirror as the object is in front of the mirror.
[Hint: CN is normal to the mirror. Also, angle of incidence = angle of reflection].
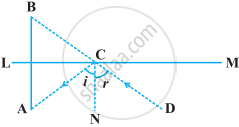
In figure, PQ ⊥ RQ, PQ = 5 cm and QR = 5 cm. Then ∆PQR is ______.