Advertisements
Advertisements
Question
The medians AD and BE of a triangle with vertices A (0, b), B (0, 0) and C (a, 0) are perpendicular to each other, if
Options
\[a = \frac{b}{2}\]
\[b = \frac{a}{2}\]
ab = 1
\[a = \pm \sqrt{2}b\]
Solution
\[a = \pm \sqrt{2}b\]
The midpoints of BC and AC are \[D\left( \frac{a}{2}, 0 \right) \text { and } E\left( \frac{a}{2}, \frac{b}{2} \right)\].
Slope of AD= \[\frac{0 - b}{\frac{a}{2} - 0}\]
Slope of BE = \[\frac{- \frac{b}{2}}{\frac{- a}{2}}\]
It is given that the medians are perpendicular to each other.
\[\frac{0 - b}{\frac{a}{2} - 0} \times \frac{- \frac{b}{2}}{- \frac{a}{2}} = - 1\]
\[ \Rightarrow a = \pm \sqrt{2}b\]
APPEARS IN
RELATED QUESTIONS
The base of an equilateral triangle with side 2a lies along they y-axis such that the mid point of the base is at the origin. Find vertices of the triangle.
Find a point on the x-axis, which is equidistant from the points (7, 6) and (3, 4).
Find the slope of a line, which passes through the origin, and the mid-point of the line segment joining the points P (0, –4) and B (8, 0).
Without using the Pythagoras theorem, show that the points (4, 4), (3, 5) and (–1, –1) are the vertices of a right angled triangle.
Without using distance formula, show that points (–2, –1), (4, 0), (3, 3) and (–3, 2) are vertices of a parallelogram.
Find the values of k for which the line (k–3) x – (4 – k2) y + k2 –7k + 6 = 0 is
- Parallel to the x-axis,
- Parallel to the y-axis,
- Passing through the origin.
Find the equation of the lines through the point (3, 2) which make an angle of 45° with the line x –2y = 3.
Find the slope of the lines which make the following angle with the positive direction of x-axis: \[\frac{\pi}{3}\]
State whether the two lines in each of the following are parallel, perpendicular or neither.
Through (9, 5) and (−1, 1); through (3, −5) and (8, −3)
State whether the two lines in each of the following is parallel, perpendicular or neither.
Through (6, 3) and (1, 1); through (−2, 5) and (2, −5)
Using the method of slope, show that the following points are collinear A (16, − 18), B (3, −6), C (−10, 6) .
Without using Pythagoras theorem, show that the points A (0, 4), B (1, 2) and C (3, 3) are the vertices of a right angled triangle.
The slope of a line is double of the slope of another line. If tangents of the angle between them is \[\frac{1}{3}\],find the slopes of the other line.
Consider the following population and year graph:
Find the slope of the line AB and using it, find what will be the population in the year 2010.
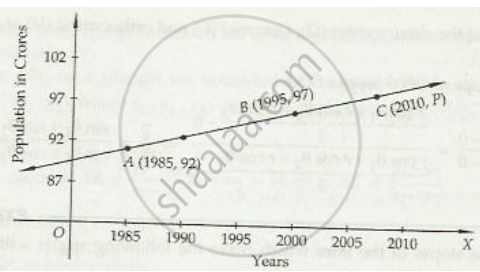
Find the value of x for which the points (x, −1), (2, 1) and (4, 5) are collinear.
Find the equation of the strainght line intersecting y-axis at a distance of 2 units above the origin and making an angle of 30° with the positive direction of the x-axis.
Find the coordinates of the orthocentre of the triangle whose vertices are (−1, 3), (2, −1) and (0, 0).
If the image of the point (2, 1) with respect to a line mirror is (5, 2), find the equation of the mirror.
Find the angles between the following pair of straight lines:
3x − y + 5 = 0 and x − 3y + 1 = 0
Find the angles between the following pair of straight lines:
x − 4y = 3 and 6x − y = 11
Find the angles between the following pair of straight lines:
(m2 − mn) y = (mn + n2) x + n3 and (mn + m2) y = (mn − n2) x + m3.
If θ is the angle which the straight line joining the points (x1, y1) and (x2, y2) subtends at the origin, prove that \[\tan \theta = \frac{x_2 y_1 - x_1 y_2}{x_1 x_2 + y_1 y_2}\text { and } \cos \theta = \frac{x_1 x_2 + y_1 y_2}{\sqrt{{x_1}^2 + {y_1}^2}\sqrt{{x_2}^2 + {y_2}^2}}\].
Show that the line a2x + ay + 1 = 0 is perpendicular to the line x − ay = 1 for all non-zero real values of a.
Find k, if the slope of one of the lines given by kx2 + 8xy + y2 = 0 exceeds the slope of the other by 6.
If x + y = k is normal to y2 = 12x, then k is ______.
The two lines ax + by = c and a′x + b′y = c′ are perpendicular if ______.
Find the equation of a straight line on which length of perpendicular from the origin is four units and the line makes an angle of 120° with the positive direction of x-axis.
If the equation of the base of an equilateral triangle is x + y = 2 and the vertex is (2, – 1), then find the length of the side of the triangle.
A variable line passes through a fixed point P. The algebraic sum of the perpendiculars drawn from the points (2, 0), (0, 2) and (1, 1) on the line is zero. Find the coordinates of the point P.
Slope of a line which cuts off intercepts of equal lengths on the axes is ______.
Equation of the line passing through (1, 2) and parallel to the line y = 3x – 1 is ______.
Equations of diagonals of the square formed by the lines x = 0, y = 0, x = 1 and y = 1 are ______.
The points A(– 2, 1), B(0, 5), C(– 1, 2) are collinear.
The three straight lines ax + by = c, bx + cy = a and cx + ay = b are collinear, if ______.
If the line joining two points A (2, 0) and B (3, 1) is rotated about A in anticlockwise direction through an angle of 15°, then the equation of the line in new position is ______.
