Advertisements
Advertisements
Question
Using the formula, sin A = `sqrt((1-cos 2A)/2) ` find the value of sin 300, it being given that cos 600 = `1/2`
Solution
A = 300
⇒ 2A = 2 × 300 = 600
By substituting the value of the given T-ratio, we get:
sin A =`sqrt((1- cos 2A)/2)`
sin `30^0 = sqrt((1-cos 60^0)/2) = sqrt(1-1/2)/2 = sqrt((1/2)/2) = sqrt(1/4) =1/2`
∴ sin `30^0 = 1/2`
APPEARS IN
RELATED QUESTIONS
If A and B are acute angles such that tan A = 1/2, tan B = 1/3 and tan (A + B) = `(tan A + tan B)/(1- tan A tan B)` A + B = ?
If sin 3θ = cos (θ – 6°) where 3θ and θ − 6° are acute angles, find the value of θ.
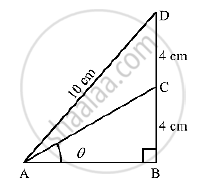
In the adjoining figure, `∠B = 90° , ∠BAC = theta° , BC = CD = 4cm and AD = 10 cm`. find (i) sin theta and (ii) `costheta`
If x = cosec A +cos A and y = cosec A – cos A then prove that `(2/(x+y))^2 + ((x-y)/2)^2` = 1
Evaluate:
`cot^2 30^0-2cos^2 30^0-3/4 sec^2 45^0 +1/4 cosec^2 30^0`
Verify each of the following:
(i)`sin 60^0 cos 30^0-cos 60^0 sin 30^0`
From the following figure, find:
(i) y
(ii) sin x°
(iii) (sec x° - tan x°) (sec x° + tan x°)

In the diagram, given below, triangle ABC is right-angled at B and BD is perpendicular to AC.
Find:
(i) cos ∠DBC
(ii) cot ∠DBA

If sec A = `sqrt2` , find : `(3cot^2 "A"+ 2 sin^2 "A")/ (tan^2 "A" – cos ^2 "A")`.
If cos A = `3/5`, then find the value of `(sin"A" - cos"A")/(2tan"A")`
