Advertisements
Advertisements
प्रश्न
Choose the correct alternative answer for the following question.
विकल्प
angle of elevation.
angle of depression.
0
straight angle.
उत्तर
When we see at a higher level, from the horizontal line, angle formed is angle of elevation.
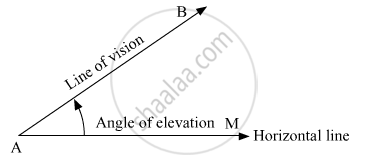
Hence, the correct answer is angle of elevation.
संबंधित प्रश्न
If \[\sin\theta = \frac{7}{25}\], find the values of cosθ and tanθ.
If 5 secθ – 12 cosecθ = 0, find the values of secθ, cosθ, and sinθ.
Prove that:
Prove that: `1/"sec θ − tan θ" = "sec θ + tan θ"`
Prove that:
Choose the correct alternative answer for the following question.
sin \[\theta\] cosec \[\theta\]= ?
Choose the correct alternative answer for the following question.
1 + tan2 \[\theta\] = ?
Prove the following.
cot2θ – tan2θ = cosec2θ – sec2θ
Prove the following.
Prove the following:
sec6x – tan6x = 1 + 3sec2x × tan2x
Prove the following.
Choose the correct alternative:
sinθ × cosecθ =?
If sinθ = `8/17`, where θ is an acute angle, find the value of cos θ by using identities.
Show that:
`sqrt((1-cos"A")/(1+cos"A"))=cos"ecA - cotA"`
ΔAMT∼ΔAHE, construct Δ AMT such that MA = 6.3 cm, ∠MAT=120°, AT = 4.9 cm and `"MA"/"HA"=7/5`, then construct ΔAHE.
Prove that: (sec θ – cos θ) (cot θ + tan θ) = tan θ.sec θ
Proof: L.H.S. = (sec θ – cos θ) (cot θ + tan θ)
= `(1/square - cos θ) (square/square + square/square)` ......`[∵ sec θ = 1/square, cot θ = square/square and tan θ = square/square]`
= `((1 - square)/square) ((square + square)/(square square))`
= `square/square xx 1/(square square)` ......`[(∵ square + square = 1),(∴ square = 1 - square)]`
= `square/(square square)`
= tan θ.sec θ
= R.H.S.
∴ L.H.S. = R.H.S.
∴ (sec θ – cos θ) (cot θ + tan θ) = tan θ.sec θ
