Advertisements
Advertisements
प्रश्न
Defects on plywood sheet occur at random with the average of one defect per 50 sq.ft. Find the probability that such a sheet has:
- no defect
- at least one defect
Use e−1 = 0.3678
उत्तर
Let X denote the number of defects on a plywood sheet.
Given, m = 1, e–1 = 0.3678
∴ X ~ P(m) ≡ X ~ P(1)
The p.m.f. of X is given by
P(X = x) = `("e"^-"m" "m"^x)/(x!)`
∴ P(X = x) = `("e"^-1 (1)^x)/(x!)`
(i) P(no defects on a plywood)
= P(X = 0)
= `("e"^-1 (1)^0)/(0!)`
= `(0.3678 xx 1)/(1)`
= 0.3678
(ii) P(at least one defect)
= P(X ≥ 1)
= 1 – P(X = 0)
= 1 – 0.3678
= 0.6322
APPEARS IN
संबंधित प्रश्न
From a lot of 25 bulbs of which 5 are defective a sample of 5 bulbs was drawn at random with replacement. Find the probability that the sample will contain -
(a) exactly 1 defective bulb.
(b) at least 1 defective bulb.
State the following are not the probability distributions of a random variable. Give reasons for your answer.
| X | 0 | 1 | 2 |
| P (X) | 0.4 | 0.4 | 0.2 |
State the following are not the probability distributions of a random variable. Give reasons for your answer.
| Z | 3 | 2 | 1 | 0 | -1 |
| P(Z) | 0.3 | 0.2 | 0.4 | 0.1 | 0.05 |
Find the probability distribution of number of heads in four tosses of a coin.
Find the probability distribution of the number of successes in two tosses of a die, where a success is defined as
(i) number greater than 4
(ii) six appears on at least one die
A coin is biased so that the head is 3 times as likely to occur as tail. If the coin is tossed twice, find the probability distribution of number of tails.
The random variable X has probability distribution P(X) of the following form, where k is some number:
`P(X = x) {(k, if x = 0),(2k, if x = 1),(3k, if x = 2),(0, "otherwise"):}`
- Determine the value of 'k'.
- Find P(X < 2), P(X ≥ 2), P(X ≤ 2).
The probability distribution function of a random variable X is given by
| xi : | 0 | 1 | 2 |
| pi : | 3c3 | 4c − 10c2 | 5c-1 |
where c > 0 Find: c
The probability distribution function of a random variable X is given by
| xi : | 0 | 1 | 2 |
| pi : | 3c3 | 4c − 10c2 | 5c-1 |
where c > 0 Find: P (X < 2)
Two cards are drawn from a well shuffled pack of 52 cards. Find the probability distribution of the number of aces.
Two dice are thrown together and the number appearing on them noted. X denotes the sum of the two numbers. Assuming that all the 36 outcomes are equally likely, what is the probability distribution of X?
A class has 15 students whose ages are 14, 17, 15, 14, 21, 19, 20, 16, 18, 17, 20, 17, 16, 19 and 20 years respectively. One student is selected in such a manner that each has the same chance of being selected and the age X of the selected student is recorded. What is the probability distribution of the random variable X?
An urn contains 4 red and 3 blue balls. Find the probability distribution of the number of blue balls in a random draw of 3 balls with replacement.
Four balls are to be drawn without replacement from a box containing 8 red and 4 white balls. If X denotes the number of red balls drawn, then find the probability distribution of X.
Find the mean and standard deviation of each of the following probability distribution:
| xi : | 1 | 3 | 4 | 5 |
| pi: | 0.4 | 0.1 | 0.2 | 0.3 |
Find the mean and standard deviation of each of the following probability distribution :
| xi : | 0 | 1 | 2 | 3 | 4 | 5 |
| pi : |
\[\frac{1}{6}\]
|
\[\frac{5}{18}\]
|
\[\frac{2}{9}\]
|
\[\frac{1}{6}\]
|
\[\frac{1}{9}\]
|
\[\frac{1}{18}\]
|
A pair of fair dice is thrown. Let X be the random variable which denotes the minimum of the two numbers which appear. Find the probability distribution, mean and variance of X.
A fair die is tossed. Let X denote 1 or 3 according as an odd or an even number appears. Find the probability distribution, mean and variance of X.
For what value of k the following distribution is a probability distribution?
| X = xi : | 0 | 1 | 2 | 3 |
| P (X = xi) : | 2k4 | 3k2 − 5k3 | 2k − 3k2 | 3k − 1 |
Find the mean of the following probability distribution:
| X= xi: | 1 | 2 | 3 |
| P(X= xi) : |
\[\frac{1}{4}\]
|
\[\frac{1}{8}\]
|
\[\frac{5}{8}\]
|
If the probability distribution of a random variable X is as given below:
Write the value of P (X ≤ 2).
| X = xi : | 1 | 2 | 3 | 4 |
| P (X = xi) : | c | 2c | 4c | 4c |
If a random variable X has the following probability distribution:
| X : | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| P (X) : | a | 3a | 5a | 7a | 9a | 11a | 13a | 15a | 17a |
then the value of a is
A random variable X has the following probability distribution:
| X : | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| P (X) : | 0.15 | 0.23 | 0.12 | 0.10 | 0.20 | 0.08 | 0.07 | 0.05 |
For the events E = {X : X is a prime number}, F = {X : X < 4}, the probability P (E ∪ F) is
Mark the correct alternative in the following question:
The probability distribution of a discrete random variable X is given below:
| X: | 2 | 3 | 4 | 5 |
| P(X): |
\[\frac{5}{k}\]
|
\[\frac{7}{k}\]
|
\[\frac{9}{k}\]
|
\[\frac{11}{k}\] |
The value of k is .
From a lot of 15 bulbs which include 5 defective, a sample of 4 bulbs is drawn one by one with replacement. Find the probability distribution of number of defective bulbs. Hence, find the mean of the distribution.
A die is tossed twice. A 'success' is getting an even number on a toss. Find the variance of number of successes.
Three cards are drawn successively with replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of spades. Hence, find the mean of the distribtution.
Five bad oranges are accidently mixed with 20 good ones. If four oranges are drawn one by one successively with replacement, then find the probability distribution of number of bad oranges drawn. Hence find the mean and variance of the distribution.
Calculate `"e"_0^circ ,"e"_1^circ , "e"_2^circ` from the following:
| Age x | 0 | 1 | 2 |
| lx | 1000 | 880 | 876 |
| Tx | - | - | 3323 |
Using the truth table verify that p ∨ (q ∧ r) ≡ (p ∨ q) ∧ (p ∨ r).
Verify the following function, which can be regarded as p.m.f. for the given values of X :
| X = x | -1 | 0 | 1 |
| P(x) | -0.2 | 1 | 0.2 |
Compute the age specific death rate for the following data :
| Age group (years) | Population (in thousands) | Number of deaths |
| Below 5 | 15 | 360 |
| 5-30 | 20 | 400 |
| Above 30 | 10 | 280 |
A fair coin is tossed 12 times. Find the probability of getting exactly 7 heads .
If random variable X has probability distribution function.
f(x) = `c/x`, 1 < x < 3, c > 0, find c, E(x) and Var(X)
A random variable X has the following probability distribution :
| X | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
| P(X) | C | 2C | 2C | 3C | C2 | 2C2 | 7C2+C |
Find the value of C and also calculate the mean of this distribution.
The p.d.f. of a continuous r.v. X is given by
f (x) = `1/ (2a)` , for 0 < x < 2a and = 0, otherwise. Show that `P [X < a/ 2] = P [X >( 3a)/ 2]` .
There are 10% defective items in a large bulk of items. What is the probability that a sample of 4 items will include not more than one defective item?
The probability that a bulb produced by a factory will fuse after 200 days of use is 0.2. Let X denote the number of bulbs (out of 5) that fuse after 200 days of use. Find the probability of X > 1
10 balls are marked with digits 0 to 9. If four balls are selected with replacement. What is the probability that none is marked 0?
In a multiple choice test with three possible answers for each of the five questions, what is the probability of a candidate getting four or more correct answers by random choice?
Solve the following problem :
A large chain retailer purchases an electric device from the manufacturer. The manufacturer indicates that the defective rate of the device is 10%. The inspector of the retailer randomly selects 4 items from a shipment. Find the probability that the inspector finds at most one defective item in the 4 selected items.
Solve the following problem :
The probability that a component will survive a check test is 0.6. Find the probability that exactly 2 of the next 4 components tested survive.
Solve the following problem :
A computer installation has 3 terminals. The probability that any one terminal requires attention during a week is 0.1, independent of other terminals. Find the probabilities that 1 terminal requires attention during a week.
Solve the following problem :
It is observed that it rains on 10 days out of 30 days. Find the probability that it rains on exactly 3 days of a week.
Find the probability distribution of the number of doublets in three throws of a pair of dice
A discrete random variable X has the probability distribution given as below:
| X | 0.5 | 1 | 1.5 | 2 |
| P(X) | k | k2 | 2k2 | k |
Find the value of k
Two biased dice are thrown together. For the first die P(6) = `1/2`, the other scores being equally likely while for the second die, P(1) = `2/5` and the other scores are equally likely. Find the probability distribution of ‘the number of ones seen’.
Let X be a discrete random variable whose probability distribution is defined as follows:
P(X = x) = `{{:("k"(x + 1), "for" x = 1"," 2"," 3"," 4),(2"k"x, "for" x = 5"," 6"," 7),(0, "Otherwise"):}`
where k is a constant. Calculate E(X)
The probability distribution of a discrete random variable X is given as under:
| X | 1 | 2 | 4 | 2A | 3A | 5A |
| P(X) | `1/2` | `1/5` | `3/25` | `1/10` | `1/25` | `1/25` |
Calculate: Variance of X
The probability distribution of a random variable x is given as under:
P(X = x) = `{{:("k"x^2, "for" x = 1"," 2"," 3),(2"k"x, "for" x = 4"," 5"," 6),(0, "otherwise"):}`
where k is a constant. Calculate P(X ≥ 4)
A random variable x has to following probability distribution.
| X | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| P(x) | 0 | k | 2k | 2k | 3k | k2 | 2k2 | 7k2 + k |
Determine
If the p.m.f of a r. v. X is
P(x) = `c/x^3`, for x = 1, 2, 3
= 0, otherwise
then E(X) = ______.
Find the mean of number randomly selected from 1 to 15.
A random variable X has the following probability distribution:
| x | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| P(x) | k | 2k | 2k | 3k | k2 | 2k2 | 7k2 + k |
Find:
- k
- P(X < 3)
- P(X > 4)
Two numbers are selected from first six even natural numbers at random without replacement. If X denotes the greater of two numbers selected, find the probability distribution of X.
The probability that a bomb will hit the target is 0.8. Complete the following activity to find, the probability that, out of 5 bombs exactly 2 will miss the target.
Solution: Here, n = 5, X =number of bombs that hit the target
p = probability that bomb will hit the target = `square`
∴ q = 1 - p = `square`
Here, `X∼B(5,4/5)`
∴ P(X = x) = `""^"n""C"_x"P"^x"q"^("n" - x) = square`
P[Exactly 2 bombs will miss the target] = P[Exactly 3 bombs will hit the target]
= P(X = 3)
=`""^5"C"_3(4/5)^3(1/5)^2=10(4/5)^3(1/5)^2`
∴ P(X = 3) = `square`
Kiran plays a game of throwing a fair die 3 times but to quit as and when she gets a six. Kiran gets +1 point for a six and –1 for any other number.
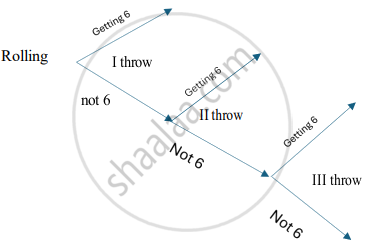
- If X denotes the random variable “points earned” then what are the possible values X can take?
- Find the probability distribution of this random variable X.
- Find the expected value of the points she gets.
