Advertisements
Advertisements
प्रश्न
Evaluate the following limit:
`lim_(z -> -5)[((1/z + 1/5))/(z + 5)]`
उत्तर
`lim_(z -> -5)[(1/z + 1/5)/(z + 5)]`
= `lim_(z -> -5) (((5 + z)/(5z)))/(z + 5)`
= `lim_(z -> - 5) 1/(5z)` ...[∵ z → – 5, z ≠ – 5 ∴ z + 5 ≠ 0]
= `(lim_(z -> - 5)(1))/(lim_(z -> - 5) (5z))`
= `1/(5( - 5))`
= `-1/25`
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit:
`lim_(x -> 2)[(x^(-3) - 2^(-3))/(x - 2)]`
Evaluate the following limit:
If `lim_(x -> 1)[(x^4 - 1)/(x - 1)]` = `lim_(x -> "a")[(x^3 - "a"^3)/(x - "a")]`, find all possible values of a
Evaluate the following limit :
`lim_(x -> 1) [(x + x^3 + x^5 + ... + x^(2"n" - 1) - "n")/(x - 1)]`
In the following example, given ∈ > 0, find a δ > 0 such that whenever, |x – a| < δ, we must have |f(x) – l| < ∈.
`lim_(x -> 2)(2x + 3)` = 7
In the following example, given ∈ > 0, find a δ > 0 such that whenever, |x – a| < δ, we must have |f(x) – l| < ∈.
`lim_(x -> 2) (x^2 - 1)` = 3
Evaluate the following :
`lim_(x -> 0)[x/(|x| + x^2)]`
Evaluate the following :
`lim_(x -> 0) {1/x^12 [1 - cos(x^2/2) - cos(x^4/4) + cos(x^2/2) cos(x^4/4)]}`
In problems 1 – 6, using the table estimate the value of the limit.
`lim_(x -> 2) (x - 2)/(x^2 - x - 2)`
| x | 1.9 | 1.99 | 1.999 | 2.001 | 2.01 | 2.1 |
| f(x) | 0.344820 | 0.33444 | 0.33344 | 0.333222 | 0.33222 | 0.332258 |
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) (sqrt(x + 3) - sqrt(3))/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.001 | 0.01 | 0.1 |
| f(x) | 0.2911 | 0.2891 | 0.2886 | 0.2886 | 0.2885 | 0.28631 |
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) sin x/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.001 | 0.01 | 0.1 |
| f(x) | 0.99833 | 0.99998 | 0.99999 | 0.99999 | 0.99998 | 0.99833 |
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) (cos x - 1)/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.0001 | 0.01 | 0.1 |
| f(x) | 0.04995 | 0.0049999 | 0.0004999 | – 0.0004999 | – 0.004999 | – 0.04995 |
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 0) sec x`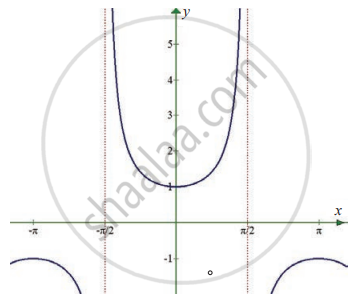
Write a brief description of the meaning of the notation `lim_(x -> 8) f(x)` = 25
If the limit of f(x) as x approaches 2 is 4, can you conclude anything about f(2)? Explain reasoning
Evaluate the following limits:
`lim_(sqrt(x) -> 3) (x^2 - 81)/(sqrt(x) - 3)`
Find the left and right limits of f(x) = `(x^2 - 4)/((x^2 + 4x+ 4)(x + 3))` at x = – 2
Evaluate the following limits:
`lim_(x -> 3) (x^2 - 9)/(x^2(x^2 - 6x + 9))`
Evaluate the following limits:
`lim_(x -> oo) 3/(x - 2) - (2x + 11)/(x^2 + x - 6)`
Evaluate the following limits:
`lim_(x -> oo) (x^4 - 5x)/(x^2 - 3x + 1)`
Evaluate the following limits:
`lim_(x -> oo) (1 + x - 3x^3)/(1 + x^2 +3x^3)`
Show that `lim_("n" -> oo) (1 + 2 + 3 + ... + "n")/(3"n"^2 + 7n" + 2) = 1/6`
Show that `lim_("n" -> oo) (1^2 + 2^2 + ... + (3"n")^2)/((1 + 2 + ... + 5"n")(2"n" + 3)) = 9/25`
Show that `lim_("n" -> oo) 1/1.2 + 1/2.3 + 1/3.4 + ... + 1/("n"("n" + 1))` = 1
A tank contains 5000 litres of pure water. Brine (very salty water) that contains 30 grams of salt per litre of water is pumped into the tank at a rate of 25 litres per minute. The concentration of salt water after t minutes (in grams per litre) is C(t) = `(30"t")/(200 + "t")`. What happens to the concentration as t → ∞?
Evaluate the following limits:
`lim_(x -> oo)(1 + "k"/x)^("m"/x)`
Evaluate the following limits:
`lim_(x -> oo) (1 + 3/x)^(x + 2)`
Evaluate the following limits:
`lim_(x -> 0) (sin("a" + x) - sin("a" - x))/x`
Evaluate the following limits:
`lim_(x -> pi) (1 + sinx)^(2"cosec"x)`
Evaluate the following limits:
`lim_(x -> 0) ("e"^x - "e"^(-x))/sinx`
Choose the correct alternative:
`lim_(x -> 0) sqrt(1 - cos 2x)/x`
Choose the correct alternative:
`lim_(theta -> 0) (sinsqrt(theta))/(sqrt(sin theta)`
Choose the correct alternative:
`lim_(x -> oo) ((x^2 + 5x + 3)/(x^2 + x + 3))^x` is
Choose the correct alternative:
`lim_(x - oo) sqrt(x^2 - 1)/(2x + 1)` =
Choose the correct alternative:
`lim_(x -> 0) (8^x - 4x - 2^x + 1^x)/x^2` =
Choose the correct alternative:
If `lim_(x -> 0) (sin "p"x)/(tan 3x)` = 4, then the value of p is
Choose the correct alternative:
`lim_(x -> 0) ("e"^tanx - "e"^x)/(tan x - x)` =
`lim_(x→∞)((x + 7)/(x + 2))^(x + 4)` is ______.
