Advertisements
Advertisements
प्रश्न
If `2^x xx3^yxx5^z=2160,` find x, y and z. Hence, compute the value of `3^x xx2^-yxx5^-z.`
उत्तर
Given `2^x xx3^yxx5^z=2160`
First, find out the prime factorisation of 2160.
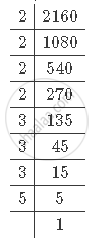
It can be observed that 2160 can be written as `2^4xx3^3xx5^1`
Also,
`2^x xx36yxx5^z=2^4xx3^3xx5^1`
⇒ x = 4, y = 3, z = 1
Therefore, the value of `3^x xx2^-yxx5^-z` is `3^4xx2^-3xx5^-1=81xx1/8xx1/5=81/40`
APPEARS IN
संबंधित प्रश्न
Simplify the following
`3(a^4b^3)^10xx5(a^2b^2)^3`
Simplify:
`root3((343)^-2)`
Prove that:
`sqrt(3xx5^-3)divroot3(3^-1)sqrt5xxroot6(3xx5^6)=3/5`
Determine `(8x)^x,`If `9^(x+2)=240+9^x`
If `3^(4x) = (81)^-1` and `10^(1/y)=0.0001,` find the value of ` 2^(-x+4y)`.
If `a=x^(m+n)y^l, b=x^(n+l)y^m` and `c=x^(l+m)y^n,` Prove that `a^(m-n)b^(n-l)c^(l-m)=1`
State the product law of exponents.
Write \[\left( \frac{1}{9} \right)^{- 1/2} \times (64 )^{- 1/3}\] as a rational number.
\[\frac{5^{n + 2} - 6 \times 5^{n + 1}}{13 \times 5^n - 2 \times 5^{n + 1}}\] is equal to
Simplify:-
`(1/3^3)^7`
