Advertisements
Advertisements
प्रश्न
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
tan C = `(5)/(12)`
उत्तर
tan C = `(5)/(12)`
tan C = `"Perpendicular"/"Base" = (5)/(12)`
By Pythagoras theprem, we have
(Hypotenuse)2 = (Perpendicular)2 + (Base)2
(Hypotenuse) = `sqrt(("Perpendicular")^2 + ("Base")^2`
= `sqrt((5)^2 + (12)^2`
= `sqrt(25 + 144)`
= `sqrt(169)`
= 13
cot C = `(1)/"tan C" = (12)/(5)`
sin C = `"Perpendicular"/"Hypotenuse" = (5)/(13)`
cos C = `"Base"/"Hypotenuse" = (12)/(13)`
sec C = `(1)/"cosC" = (13)/(12)`
cosec C = `(1)/"sin C" = (13)/(5)`.
APPEARS IN
संबंधित प्रश्न
Using the formula, sin A = `sqrt((1-cos 2A)/2) ` find the value of sin 300, it being given that cos 600 = `1/2`
If cot θ= 1; find the value of: 5 tan2 θ+ 2 sin2 θ- 3
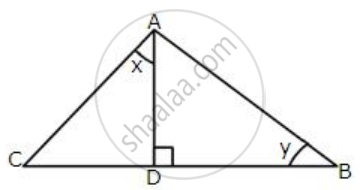
In the following figure:
AD ⊥ BC, AC = 26 CD = 10, BC = 42, ∠DAC = x and ∠B = y.
Find the value of :
(i) cot x
(ii) `1/sin^2 y – 1/tan^2 y`
(iii) `6/cos x – 5/cos y + 8 tan y`.
In triangle ABC; ∠ABC = 90°, ∠CAB = x°, tan x° = `(3)/(4)` and BC = 15 cm. Find the measures of AB and AC.
In the given figure; ∠C = 90o and D is mid-point of AC.
Find :
(i) `(tan∠CAB)/ (tan∠CDB)` (ii) `(tan∠ABC)/ (tan∠DBC)`
If 5 cos θ = 3, evaluate : `(co secθ – cot θ)/(co secθ + cot θ)`
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: cos C
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: cot C
If 8 tanθ = 15, find (i) sinθ, (ii) cotθ, (iii) sin2θ - cot2θ
From the given figure, find the values of tan C
