Advertisements
Advertisements
प्रश्न
In the given figure: AB//FD, AC//GE and BD = CE;
prove that:
- BG = DF
- CF = EG
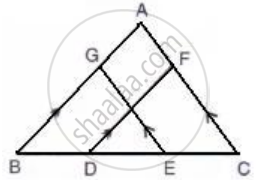
उत्तर
In the given figure AB || FD,
⇒ ∠ABC =∠FDC
Also AC || GE,
⇒ ∠ACB = ∠GEB
Consider the two triangles ΔGBE and ΔFDC
∠B = ∠D ...(Corresponding angle)
∠C = ∠E ...(Corresponding angle)
Also given that
BD = CE
⇒ BD + DE = CE + DE
⇒ BE = DC
∴ By Angle-Side-Side-Angle criterion of congruence
ΔGBE ≅ ΔFDC
∴ `"GB"/"FD" = "BE"/"DC" = "GE"/"FC"`
But BE = DC
⇒ `"BE"/"DC" = "BE"/"BE"` = 1
∴ `"GB"/"FD" = "BE"/"DC"` = 1
⇒ GB = FD
∴ `"GE"/"FC" = "BE"/"DC"` = 1
⇒ GE = FC
APPEARS IN
संबंधित प्रश्न
If perpendiculars from any point within an angle on its arms are congruent, prove that it lies on the bisector of that angle.
Prove that the perimeter of a triangle is greater than the sum of its altitudes.
In two congruent triangles ABC and DEF, if AB = DE and BC = EF. Name the pairs of equal angles.
If AP bisects angle BAC and M is any point on AP, prove that the perpendiculars drawn from M to AB and AC are equal.
In the following figure, BL = CM.

Prove that AD is a median of triangle ABC.
In the figure, given below, triangle ABC is right-angled at B. ABPQ and ACRS are squares. 
Prove that:
(i) ΔACQ and ΔASB are congruent.
(ii) CQ = BS.
A point O is taken inside a rhombus ABCD such that its distance from the vertices B and D are equal. Show that AOC is a straight line.
In the following figure, AB = EF, BC = DE and ∠B = ∠E = 90°.
Prove that AD = FC.
In ΔABC, AB = AC and the bisectors of angles B and C intersect at point O.
Prove that : (i) BO = CO
(ii) AO bisects angle BAC.
In the following figure, ABC is an equilateral triangle in which QP is parallel to AC. Side AC is produced up to point R so that CR = BP.
Prove that QR bisects PC.
Hint: ( Show that ∆ QBP is equilateral
⇒ BP = PQ, but BP = CR
⇒ PQ = CR ⇒ ∆ QPM ≅ ∆ RCM ).
