Advertisements
Advertisements
प्रश्न
ΔRHP ~ ΔNED, In ΔNED, NE = 7 cm, ∠D = 30°, ∠N = 20° and `"HP"/"ED" = 4/5`. Then construct ΔRHP and ΔNED
उत्तर

In ∆NED, ∠D = 30°
and ∠N = 20° ......(i) [Given]
∴ ∠E = 130° .....(ii) [Remaining angle of a triangle]
∆RHP ∼ ∆NED
∴ `"RH"/"NE" = "HP"/"ED" = "PR"/"DN"` .....[Corresponding sides of similar triangles]
∴ `"RH"/7 = 4/5` ......[Given]
∴ RH = `(4 xx 7)/5` = 5.6 cm
Also, ∠R = ∠N, ∠H = ∠E, ∠P = ∠D ......(iii) [Corresponding angles of similar triangles]
∴ ∠R = 20°, ∠H = 130°, ∠P = 30° ......[From (i),(ii) and (iii)]

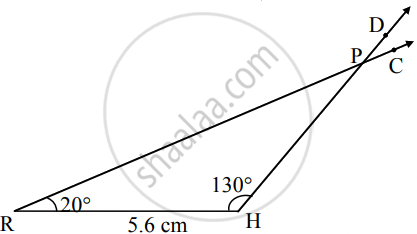
Steps of Construction:
| ∆NED | ∆RHP | |
| i. | Draw seg NE of 7 cm | Draw seg RH of 5.6 cm |
| ii. | Draw a ray NA and EB such that ∠ANE = 20° and ∠BEN = 130°. | Draw a ray RC and HD such that ∠CRH = 20° and ∠DHR = 130°. |
| iii. | Name the point of intersection of rays D. | Name the point of intersection of rays P. |
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC in which AB = 5 cm, BC = 6 cm and ∠ABC = 60˚. Now construct another triangle whose sides are 5/7 times the corresponding sides of ΔABC.
Construct an isosceles triangle with base 8 cm and altitude 4 cm. Construct another triangle whose sides are `2/3` times the corresponding sides of the isosceles triangle.
Draw a right triangle in which the sides (other than the hypotenuse) are of lengths 4 cm and 3 cm. Now construct another triangle whose sides are `3/5` times the corresponding sides of the given triangle.
Divide a line segment of length 9 cm internally in the ratio 4 : 3. Also, give justification of the construction.
Draw a ΔABC in which BC = 6 cm, AB = 4 cm and AC = 5 cm. Draw a triangle similar to ΔABC with its sides equal to (3/4)th of the corresponding sides of ΔABC.
Construct a ΔABC in which AB = 5 cm. ∠B = 60° altitude CD = 3cm. Construct a ΔAQR similar to ΔABC such that side ΔAQR is 1.5 times that of the corresponding sides of ΔACB.
Construct an isosceles triangle whose base is 8 cm and altitude 4 cm and then another triangle whose sides are 3/2 times the corresponding sides of the isosceles triangle.
Construct a triangle similar to ΔABC in which AB = 4.6 cm, BC = 5.1 cm, ∠A = 60° with scale factor 4 : 5.
Draw a line segment AB of length 7 cm. Using ruler and compasses, find a point P on AB such that `(AP)/(AB) = 3/5 `.
Draw a line segment of length 7.6 cm and divide it in the ratio 5:8. Measure the two parts.
Draw a ∆ABC in which AB = 4 cm, BC = 5 cm and AC = 6 cm. Then construct another triangle whose sides are\[\frac{3}{5}\] of the corresponding sides of ∆ABC ?
Draw a triangle ABC with side BC = 6 cm, ∠C = 30° and ∠A = 105°. Then construct another triangle whose sides are `2/3` times the corresponding sides of ΔABC.
∆PQR ~ ∆LTR. In ∆PQR, PQ = 4.2 cm, QR = 5.4 cm, PR = 4.8 cm. Construct ∆PQR and ∆LTR, such that `"PQ"/"LT" = 3/4`.
If A (20, 10), B(0, 20) are given, find the coordinates of the points which divide segment AB into five congruent parts.
Draw a line segment AB of length 7 cm. Using ruler and compasses, find a point P on AB such that `(AP)/(AB)=3/5`.
Draw a right triangle in which the sides (other than the hypotenuse) are of lengths 4 cm and 3 cm. Now construct another triangle whose sides are \[\frac{3}{5}\] times the corresponding sides of the given triangle.
Δ AMT ∼ ΔAHE. In Δ AMT, MA = 6.3 cm, ∠MAT = 120°, AT = 4.9 cm, `(MA)/(HA) = 7/5`. construct Δ AHE.
Choose the correct alternative:

In the figure ΔABC ~ ΔADE then the ratio of their corresponding sides is ______
∆ABC ~ ∆PBQ. In ∆ABC, AB = 3 cm, ∠B = 90°, BC = 4 cm. Ratio of the corresponding sides of two triangles is 7 : 4. Then construct ∆ABC and ∆PBQ
ΔRHP ~ ΔNED, In ΔNED, NE = 7 cm. ∠D = 30°, ∠N = 20°, `"HP"/"ED" = 4/5`, then construct ΔRHP and ∆NED
ΔABC ~ ΔPBR, BC = 8 cm, AC = 10 cm , ∠B = 90°, `"BC"/"BR" = 5/4` then construct ∆ABC and ΔPBR
To divide a line segment AB in the ratio p : q (p, q are positive integers), draw a ray AX so that ∠BAX is an acute angle and then mark points on ray AX at equal distances such that the minimum number of these points is ______.
To construct a triangle similar to a given ΔABC with its sides `3/7` of the corresponding sides of ΔABC, first draw a ray BX such that ∠CBX is an acute angle and X lies on the opposite side of A with respect to BC. Then locate points B1, B2, B3, ... on BX at equal distances and next step is to join ______.
A triangle ABC is such that BC = 6cm, AB = 4cm and AC = 5cm. For the triangle similar to this triangle with its sides equal to `3/4`th of the corresponding sides of ΔABC, correct figure is?
To divide a line segment PQ in the ratio 5 : 7, first a ray PX is drawn so that ∠QPX is an acute angle and then at equal distances points are marked on the ray PX such that the minimum number of these points is ______.
When a line segment is divided in the ratio 2 : 3, how many parts is it divided into?
If I ask you to construct ΔPQR ~ ΔABC exactly (when we say exactly, we mean the exact relative positions of the triangles) as given in the figure, (Assuming I give you the dimensions of ΔABC and the Scale Factor for ΔPQR) what additional information would you ask for?
If you need to construct a triangle with point P as one of its vertices, which is the angle that you need to construct a side of the triangle?

The image of construction of A’C’B a similar triangle of ΔACB is given below. Then choose the correct option.
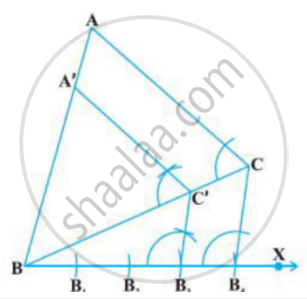
If a triangle similar to given ΔABC with sides equal to `3/4` of the sides of ΔABC is to be constructed, then the number of points to be marked on ray BX is ______.

The basic principle used in dividing a line segment is ______.
Draw a right triangle ABC in which BC = 12 cm, AB = 5 cm and ∠B = 90°. Construct a triangle similar to it and of scale factor `2/3`. Is the new triangle also a right triangle?
Draw a triangle ABC in which BC = 6 cm, CA = 5 cm and AB = 4 cm. Construct a triangle similar to it and of scale factor `5/3`.
Draw a triangle ABC in which AB = 5 cm, BC = 6 cm and ∠ABC = 60°. Construct a triangle similar to ∆ABC with scale factor `5/7`. Justify the construction.
Draw a line segment of length 7.5 cm and divide it in the ratio 1:3.
